
State and prove the Pythagoras theorem.
Answer
522.9k+ views
37 likes
Hint: Draw a perpendicular on AC from B and use angle-angle similarity to prove the theorem.
Complete step-by-step answer:
Pythagoras theorem states that “ In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides”.
The sides of the right-angled triangle are called base, perpendicular and hypotenuse .
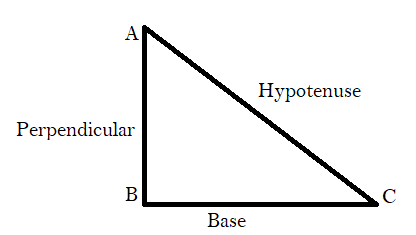
According to Pythagoras theorem ,
Proof:
Given, a triangle ABC in which
Construction: Draw a perpendicular BD on AC i.e. BD
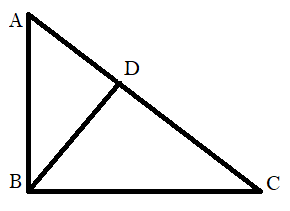
In
Therefore
So,
In
Therefore
So,
Adding equation (1) and (2) , we get
Hence, proved.
Note: In a right angled triangle , hypotenuse is the longest side of the triangle and is opposite to the right angle i.e.
Complete step-by-step answer:
Pythagoras theorem states that “ In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides”.
The sides of the right-angled triangle are called base, perpendicular and hypotenuse .

According to Pythagoras theorem ,
Proof:
Given, a triangle ABC in which
Construction: Draw a perpendicular BD on AC i.e. BD

In
Therefore
So,
In
Therefore
So,
Adding equation (1) and (2) , we get
Hence, proved.
Note: In a right angled triangle , hypotenuse is the longest side of the triangle and is opposite to the right angle i.e.
Latest Vedantu courses for you
Grade 6 | ICSE | SCHOOL | English
Vedantu ICSE 6 Pro Course (2025-26)
School Full course for ICSE students
₹35,200 per year
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




