
State and derive theorem for parallel and perpendicular axes.
Answer
446.2k+ views
6 likes
Hint
According to the perpendicular axis theorem for an axis perpendicular to the plane, the moment of inertia is equal to the sum of the moments of inertia about 2 mutually perpendicular axes in its own plane. And according to the parallel axis theorem, the moment of inertia about any axis is equal to sum of parallel and passing through the center of mass and product of mass of body and square of distance between the axes.
Complete step by step answer
The parallel axis theorem states that, the moment of inertia of a body about any axis is equal to the moment of inertia about parallel axis through its center of mass plus the product of the mass of the body and the square of the perpendicular distance between the two parallel axes.
Let us consider
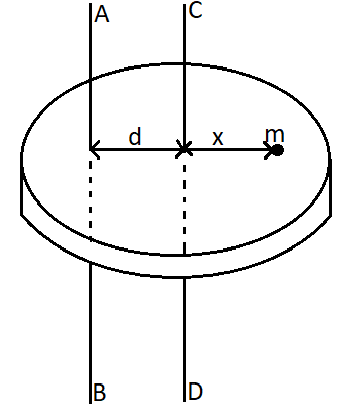
Let us consider a particle of mass
So the moment of inertia of this particle about the axis AB is
So to get the moment of inertia about the whole body we use summation. Therefore, we get
Now we can break the whole square in the formula as,
So on breaking the individual terms under the summation we get
Now we can write
Since the value of
For the third term,
Therefore, substituting the values we get
This is the parallel axis theorem.
The perpendicular axis theorem states that the moment of inertia of a plane lamina about a perpendicular axis is equal to the sum of the moments of inertia of the lamina about any 2 mutually perpendicular axes in its own plane and intersecting each other at the point where the perpendicular axis passes through the lamina.
Let us consider another plane lamina as in the figure.
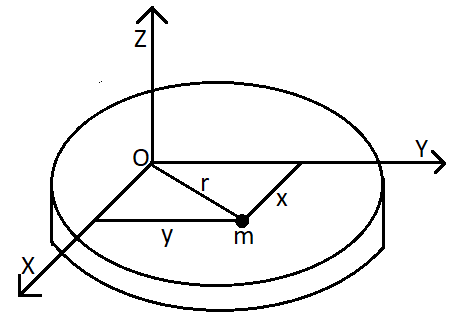
We consider a particle of mass
Therefore from the figure we can write,
So the moment of inertia of the particle about X-axis is
Therefore, for the whole bode, we consider summation and get moment of inertia as,,
Similarly moment of inertia of the whole body about Y-axis is
And the moment of inertia about Z-axis is
We can substitute
On opening the bracket we get
We can write this as,
This is the perpendicular axes theorem.
Note
The parallel and perpendicular axes theorem are used to find the moment of inertia of various rigid structures about different axes like a rod, disc, sphere, ring, etc. It gives us a simplified way of finding the moment of inertia.
According to the perpendicular axis theorem for an axis perpendicular to the plane, the moment of inertia is equal to the sum of the moments of inertia about 2 mutually perpendicular axes in its own plane. And according to the parallel axis theorem, the moment of inertia about any axis is equal to sum of parallel and passing through the center of mass and product of mass of body and square of distance between the axes.
Complete step by step answer
The parallel axis theorem states that, the moment of inertia of a body about any axis is equal to the moment of inertia about parallel axis through its center of mass plus the product of the mass of the body and the square of the perpendicular distance between the two parallel axes.
Let us consider

Let us consider a particle of mass
So the moment of inertia of this particle about the axis AB is
So to get the moment of inertia about the whole body we use summation. Therefore, we get
Now we can break the whole square in the formula as,
So on breaking the individual terms under the summation we get
Now we can write
Since the value of
For the third term,
Therefore, substituting the values we get
This is the parallel axis theorem.
The perpendicular axis theorem states that the moment of inertia of a plane lamina about a perpendicular axis is equal to the sum of the moments of inertia of the lamina about any 2 mutually perpendicular axes in its own plane and intersecting each other at the point where the perpendicular axis passes through the lamina.
Let us consider another plane lamina as in the figure.

We consider a particle of mass
Therefore from the figure we can write,
So the moment of inertia of the particle about X-axis is
Therefore, for the whole bode, we consider summation and get moment of inertia as,,
Similarly moment of inertia of the whole body about Y-axis is
And the moment of inertia about Z-axis is
We can substitute
On opening the bracket we get
We can write this as,
This is the perpendicular axes theorem.
Note
The parallel and perpendicular axes theorem are used to find the moment of inertia of various rigid structures about different axes like a rod, disc, sphere, ring, etc. It gives us a simplified way of finding the moment of inertia.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




