
How do you solve
Answer
469.5k+ views
Hint: In the problem they have mentioned to use the method of completing the square. We know that the method of completing the square is used to solve or find the roots of a quadratic equation. So first we will check whether the given equation is a quadratic equation or not. If the given equation is a quadratic equation and which is in the form of
Complete step by step solution:
Given equation,
We can clearly see that the above equation is a quadratic equation, so we can use the method of completing the square to solve the given equation.
Comparing the given equation with
We have the value
In the above equation the coefficient of
Rearranging and rewriting the terms in the above equation, then we will get
We know that
Taking the constants in the above equation to the other side of the equation, then we will get
Applying square root on both side of the above equation, then we will get
From the above equation, we will get
Simplifying the above equations, then
Hence the roots of the given equation
Note: We can also plot the graph of the given equation to get the roots of the given equation. When we plot the graph of the equation
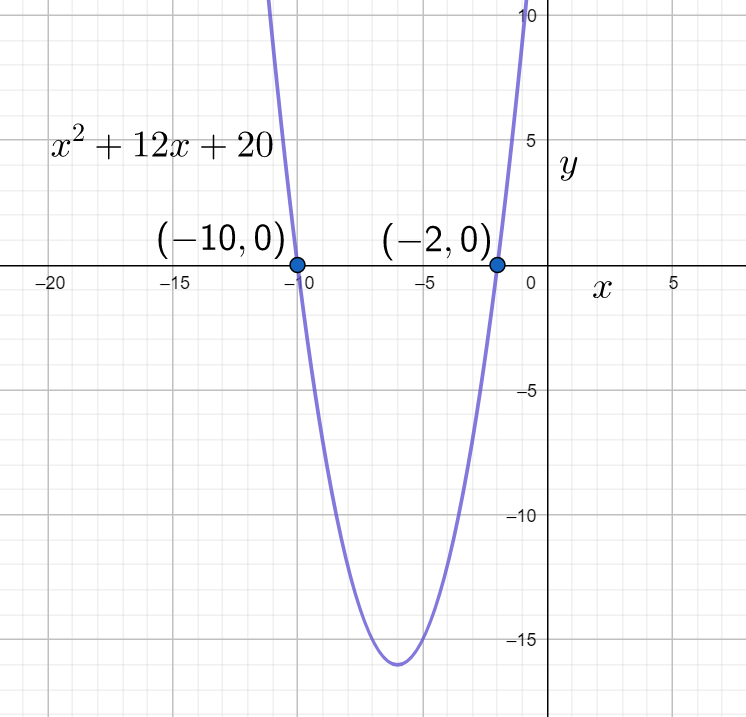
From the above graph also, we can write the roots of the given equation
Complete step by step solution:
Given equation,
We can clearly see that the above equation is a quadratic equation, so we can use the method of completing the square to solve the given equation.
Comparing the given equation with
We have the value
In the above equation the coefficient of
Rearranging and rewriting the terms in the above equation, then we will get
We know that
Taking the constants in the above equation to the other side of the equation, then we will get
Applying square root on both side of the above equation, then we will get
From the above equation, we will get
Simplifying the above equations, then
Hence the roots of the given equation
Note: We can also plot the graph of the given equation to get the roots of the given equation. When we plot the graph of the equation

From the above graph also, we can write the roots of the given equation
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

A car travels 100 km at a speed of 60 kmh and returns class 11 physics CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE

In tea plantations and hedge making gardeners trim class 11 biology CBSE

A difference between diffusion and osmosis is a A semipermeable class 11 chemistry CBSE




