
How do you solve and graph the absolute value inequality
Answer
475.2k+ views
Hint: To solve the inequality given in the above problem is as follows:
Complete step-by-step solution:
The inequality of modulus form given in the above problem is as follows:
Now, we are going to solve the above inequality by opening this modulus form and we get,
Solving the above two inequalities one by one and then taking union of these two inequality solutions.
Solving the first inequality in the above we get,
Subtracting 4 on both the sides in the above expression we get,
Dividing 2 on both the sides we get,
Solving the second inequality we get,
Subtracting 4 on both the sides we get,
Dividing 2 on both the sides we get,
Now, taking union of the solution of the above two inequalities we get,
Now, converting the above inequalities into interval notation we get,
Converting
Converting
Now, union of the above two intervals are as follows:
Hence, the solution of the inequality given above is as follows:
Now, we are going to draw the graph of the above inequality. For that, first of all, we are going to draw the graph of the modulus function.
The function written in the modulus is as follows:
The graph of this function is as follows:
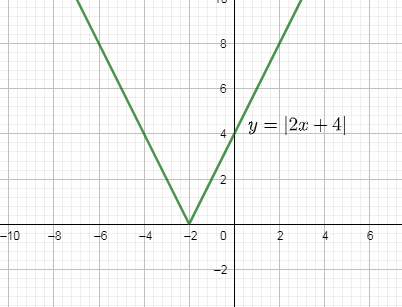
Now, in the above problem we need the values when y takes value greater than 8 so we will eliminate the area in the above graph where y’s value is less than or equal to 8.
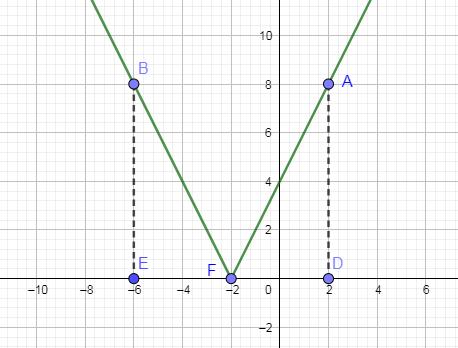
In the above graph, as you can see that when the value of function is 8 then two x values are coming i.e. (2 and -6) so we are removing the portion BFA from the above graph to get the required graph.
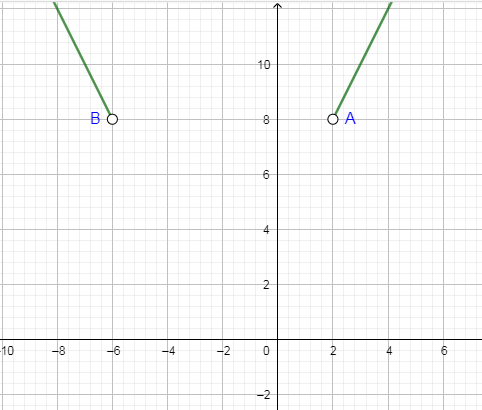
The open holes of points A and B are suggesting that at x equals -6 and 2 the y is not taking a value. Hence, we have drawn the graph of
Note: As you can see that the graph we have drawn above lies in the same interval in which we have calculated the solution for the inequality. The solution of the inequality
And the graph which we have drawn above is as follows:

Now, you can see that in the above graph the value of x lies from negative infinity to -6( in which -6 is not included) and from 2 to infinity (in which 2 is not included).
So, basically, graphical representation is a proof that the solution which we have found is correct.
Complete step-by-step solution:
The inequality of modulus form given in the above problem is as follows:
Now, we are going to solve the above inequality by opening this modulus form and we get,
Solving the above two inequalities one by one and then taking union of these two inequality solutions.
Solving the first inequality in the above we get,
Subtracting 4 on both the sides in the above expression we get,
Dividing 2 on both the sides we get,
Solving the second inequality we get,
Subtracting 4 on both the sides we get,
Dividing 2 on both the sides we get,
Now, taking union of the solution of the above two inequalities we get,
Now, converting the above inequalities into interval notation we get,
Converting
Converting
Now, union of the above two intervals are as follows:
Hence, the solution of the inequality given above is as follows:
Now, we are going to draw the graph of the above inequality. For that, first of all, we are going to draw the graph of the modulus function.
The function written in the modulus is as follows:
The graph of this function is as follows:

Now, in the above problem we need the values when y takes value greater than 8 so we will eliminate the area in the above graph where y’s value is less than or equal to 8.

In the above graph, as you can see that when the value of function is 8 then two x values are coming i.e. (2 and -6) so we are removing the portion BFA from the above graph to get the required graph.

The open holes of points A and B are suggesting that at x equals -6 and 2 the y is not taking a value. Hence, we have drawn the graph of
Note: As you can see that the graph we have drawn above lies in the same interval in which we have calculated the solution for the inequality. The solution of the inequality
And the graph which we have drawn above is as follows:

Now, you can see that in the above graph the value of x lies from negative infinity to -6( in which -6 is not included) and from 2 to infinity (in which 2 is not included).
So, basically, graphical representation is a proof that the solution which we have found is correct.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




