
Sketch the graph for
Answer
492.9k+ views
Hint:
To sketch the graph of
In a relation R from set A to set B, the set of all first components of order pair belongings to R is called the domain and all the second element of pair is called range.
Complete step by step solution:
We know that the domain of tan. function(tangent function) is the set
here R represents the real number and
The range of the tan function in R. Here R represents the real number. If we restrict the domain of a tangent function to
The graph of a tan function
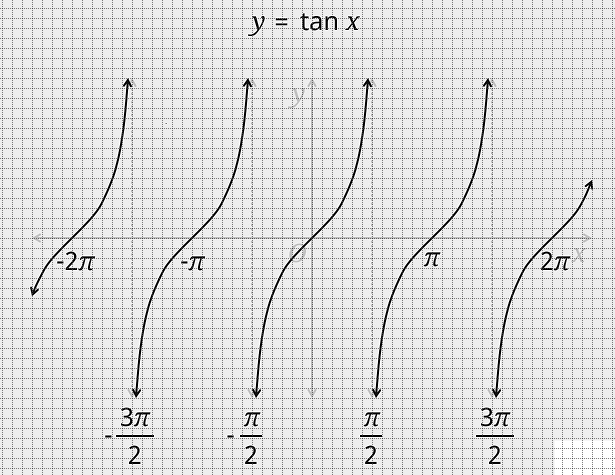
The graph of

As
As we know that
This has been defined for
Thus this graph of y where y is not defined for
Note:
One-one function: A function
Onto function: A function is
Bijective Function: The function which is both one-one and onto is called the bijective function.
To sketch the graph of
In a relation R from set A to set B, the set of all first components of order pair belongings to R is called the domain and all the second element of pair is called range.
Complete step by step solution:
We know that the domain of tan. function(tangent function) is the set
here R represents the real number and
The range of the tan function in R. Here R represents the real number. If we restrict the domain of a tangent function to
The graph of a tan function
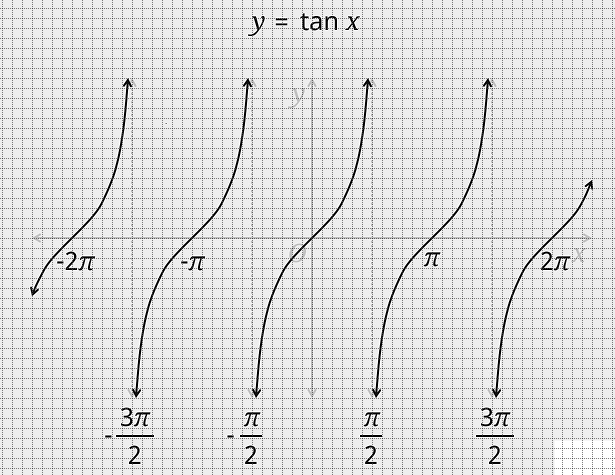
The graph of

As
As we know that
This has been defined for

Thus this graph of y where y is not defined for
Note:
One-one function: A function
Onto function: A function is
Bijective Function: The function which is both one-one and onto is called the bijective function.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




