
Prove that
Answer
518.1k+ views
1 likes
Hint: We break the angle values inside the bracket in such a way so we can relate to the quadrants in the plane and write the values for the question in a simpler way. Try to break the values as adding or subtracting from
* We know the values of all trigonometric angles are positive in the first quadrant.
Values of only
Values of only
Values of only
Complete step-by-step answer:
We solve for all the trigonometric terms separately.
For better understanding we draw the quadrant division
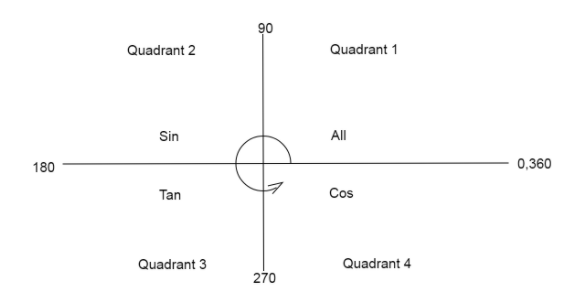
Firstly we solve for
We can write
Since
Now we can write
Since
So,
Now we solve for
We can write
Since
Now we can write
Since
So,
Now we solve for
Since,
We can write
Since
Since,
So,
Now we solve for
Since,
We can write
Since
So,
Substitute values from equation (1), (2), (3) and (4) in the following equation
Therefore, LHS=RHS
Hence Proved
Note: Students can many times make mistakes when the angle between the brackets is negative, so always check first if the trigonometric function alone is an even or odd function. An odd function brings out the negative sign while an even function eradicates the negative sign.
* We know the values of all trigonometric angles are positive in the first quadrant.
Values of only
Values of only
Values of only
Complete step-by-step answer:
We solve for all the trigonometric terms separately.
For better understanding we draw the quadrant division

Firstly we solve for
We can write
Since
Now we can write
Since
So,
Now we solve for
We can write
Since
Now we can write
Since
So,
Now we solve for
Since,
We can write
Since
Since,
So,
Now we solve for
Since,
We can write
Since
So,
Substitute values from equation (1), (2), (3) and (4) in the following equation
Therefore, LHS=RHS
Hence Proved
Note: Students can many times make mistakes when the angle between the brackets is negative, so always check first if the trigonometric function alone is an even or odd function. An odd function brings out the negative sign while an even function eradicates the negative sign.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

A car travels 100 km at a speed of 60 kmh and returns class 11 physics CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE

In tea plantations and hedge making gardeners trim class 11 biology CBSE

A difference between diffusion and osmosis is a A semipermeable class 11 chemistry CBSE




