
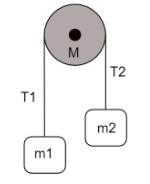
On a pulley of mass
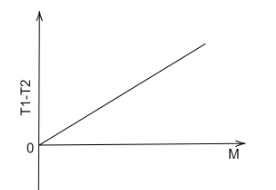
(A)
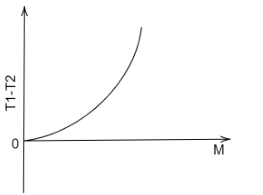
(B)
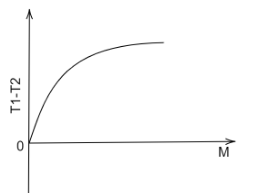
(C)
(D)





Answer
472.5k+ views
Hint: In order to find the difference, we first draw the free body diagrams of both masses, and balance the forces. We then add both of these equations, this is the first equation. The torque on the pulley is then equated with the tension in the rope, which gives rise to a second equation. Both of these equations are then combined to define the relation of
Complete step by step solution
It is given in the question that the pulley rotates without friction and the rope connecting the masses
It is also mentioned that
As they both are connected via rope, the acceleration of both blocks would be the same, but in opposite directions.
Therefore drawing the free body diagrams of both masses assuming these conditions,
For block
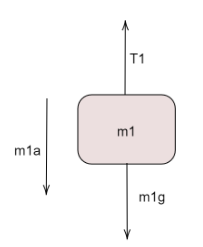
Balancing the vertical forces and acceleration,
For block
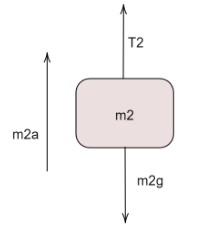
Balancing the vertical forces and acceleration,
Adding equations (a) and (b), we obtain-
On rearranging this,
Now, on drawing the free body diagram of the pulley,

We see an angular acceleration of the pulley, which is caused by the turning of the rope around it, therefore the linear component of this acceleration must be equal to the torque produced by the rope.
(The difference in the tensile forces is used as the torque to produce rotation in the pulley.)
Also,
where
For a circular disk, the Moment of inertia about its central axis of rotation is given by,
Keeping the mass of the pulley equal to
The torque is,
On equating (c) and (d) we get,
Writing in terms of linear acceleration using the relation,
we have,
Substituting the value of
Dividing both sides with
Upon cross multiplying we get,
As the value of
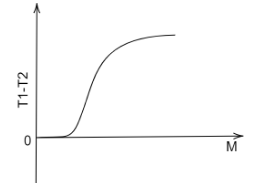
Therefore, the graph which best represents this relation is option (C), hence it is the answer.
Note
For a fixed value of
Complete step by step solution
It is given in the question that the pulley rotates without friction and the rope connecting the masses
It is also mentioned that
As they both are connected via rope, the acceleration of both blocks would be the same, but in opposite directions.
Therefore drawing the free body diagrams of both masses assuming these conditions,
For block

Balancing the vertical forces and acceleration,
For block

Balancing the vertical forces and acceleration,
Adding equations (a) and (b), we obtain-
On rearranging this,
Now, on drawing the free body diagram of the pulley,

We see an angular acceleration of the pulley, which is caused by the turning of the rope around it, therefore the linear component of this acceleration must be equal to the torque produced by the rope.
(The difference in the tensile forces is used as the torque to produce rotation in the pulley.)
Also,
where
For a circular disk, the Moment of inertia about its central axis of rotation is given by,
Keeping the mass of the pulley equal to
The torque is,
On equating (c) and (d) we get,
Writing in terms of linear acceleration using the relation,
we have,
Substituting the value of
Dividing both sides with
Upon cross multiplying we get,
As the value of
Therefore, the graph which best represents this relation is option (C), hence it is the answer.
Note
For a fixed value of
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹31,500 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




