
Obtain an expression for the rise of a liquid in a capillary tube.
Answer
487.8k+ views
Hint: A liquid can climb up the capillary tube due to the surface tension. The factors that determine the height of the fluid raised inside a capillary tube are the surface tension of the liquid, the radius of the capillary tube, the curvature of the meniscus, density of the liquid, and the acceleration due to gravity.
Complete step by step solution
Consider a capillary tube or radius
The lower end of the tube is in contact with water while its upper end is in contact with air, therefore liquid only rises to a definite height
When the liquid rises, it forms an upward concave shaped meniscus. And thus the angle made by the vertical and tangent through the meniscus is
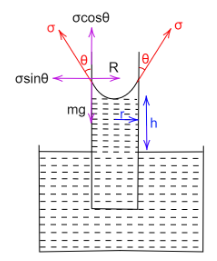
The weight of the liquid that rises in the tube is given by,
where
We know that the density is given by,
And the volume of the liquid that rises can be given by,
By substituting all these values in the formula of weight,
We obtain the weight of the liquid that rises as,
Now, the surface tension acts tangentially to the surface of the concave meniscus that is formed, therefore the tensile force in the vertical direction is given by,
When the liquid rises up to its full height, the vertical component of the surface tension and the weight of the liquid balance each other.
Therefore, equating both of these values, we have-
Therefore the expression for the rise of liquid is obtained.
Note
A liquid only rises in a capillary tube if it is a wetting liquid. The adhesive forces dominate the cohesive forces in a wetting liquid. The liquid particles tend to be attracted more to the surface of the container as compared to their own molecules, thus the liquid climbs up the capillary tube.
Complete step by step solution
Consider a capillary tube or radius
The lower end of the tube is in contact with water while its upper end is in contact with air, therefore liquid only rises to a definite height
When the liquid rises, it forms an upward concave shaped meniscus. And thus the angle made by the vertical and tangent through the meniscus is

The weight of the liquid that rises in the tube is given by,
where
We know that the density is given by,
And the volume of the liquid that rises can be given by,
By substituting all these values in the formula of weight,
We obtain the weight of the liquid that rises as,
Now, the surface tension acts tangentially to the surface of the concave meniscus that is formed, therefore the tensile force in the vertical direction is given by,
When the liquid rises up to its full height, the vertical component of the surface tension and the weight of the liquid balance each other.
Therefore, equating both of these values, we have-
Therefore the expression for the rise of liquid is obtained.
Note
A liquid only rises in a capillary tube if it is a wetting liquid. The adhesive forces dominate the cohesive forces in a wetting liquid. The liquid particles tend to be attracted more to the surface of the container as compared to their own molecules, thus the liquid climbs up the capillary tube.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




