
Mention the expression for velocity and acceleration of a particle executing SHM.
Answer
444.3k+ views
Hint:To find the expressions of velocity and acceleration of a particle executing simple harmonic motion, we need to use the equation of displacement of the particle from its equilibrium position in simple harmonic motion. The first derivative of the displacement with respect to time will give us the expression of velocity and by further differentiation, the second derivative of the displacement with respect to time will give us the expression of acceleration.
Formulas used:
Where,
Where,
Where,
Complete step by step answer:
We will first derive the expression for the velocity of the particle which executes simple harmonic motion by differentiating its displacement from the equilibrium position with respect to time. We know that the displacement from equilibrium position is given by:
Now, at the equilibrium, initial phase angle is zero which means
Also at equilibrium,
Putting this value in the equation of velocity, we get
Thus, the expression of the velocity of the particle executes simple harmonic motion is
Now, let us find the expression of acceleration of the particle executing simple harmonic motion by differentiating its velocity with respect to time.
But, we know that
Thus, the expressions for velocity and acceleration of a particle executing simple harmonic motion are
Additional information:
Squaring both sides, we get
This is the equation of an ellipse.
Therefore, we can say that the curve between displacement and velocity of a particle executing the simple harmonic motion is an ellipse.
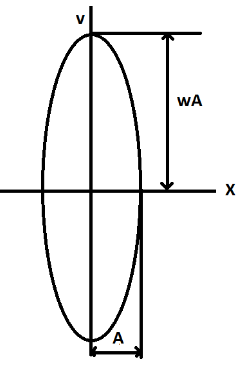
Also, the acceleration of the particle in simple harmonic motion is given by
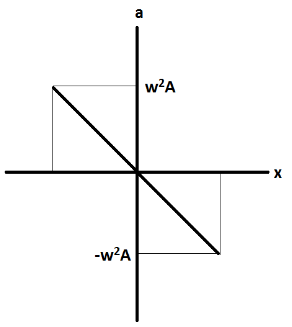
Note:Here, we have seen that the displacement of the particle executing SHM is dependent on amplitude, angular frequency, time and initial face angle. We have derived the formula for velocity and acceleration of this particle using its displacement. That is why they both are also dependent on the same parameters.
Formulas used:
Where,
Where,
Where,
Complete step by step answer:
We will first derive the expression for the velocity of the particle which executes simple harmonic motion by differentiating its displacement from the equilibrium position with respect to time. We know that the displacement from equilibrium position is given by:
Now, at the equilibrium, initial phase angle is zero which means
Also at equilibrium,
Putting this value in the equation of velocity, we get
Thus, the expression of the velocity of the particle executes simple harmonic motion is
Now, let us find the expression of acceleration of the particle executing simple harmonic motion by differentiating its velocity with respect to time.
But, we know that
Thus, the expressions for velocity and acceleration of a particle executing simple harmonic motion are
Additional information:
Squaring both sides, we get
This is the equation of an ellipse.
Therefore, we can say that the curve between displacement and velocity of a particle executing the simple harmonic motion is an ellipse.

Also, the acceleration of the particle in simple harmonic motion is given by

Note:Here, we have seen that the displacement of the particle executing SHM is dependent on amplitude, angular frequency, time and initial face angle. We have derived the formula for velocity and acceleration of this particle using its displacement. That is why they both are also dependent on the same parameters.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




