
What is meant by polar form and rectangular form ? How do you find them for complex equations?
Answer
411.3k+ views
Hint:In this question, we need to explain what polar form and rectangular form is .Mathematically, There are two basic forms of complex number notation namely polar and rectangular. To answer this kind of problem we need to know the concept of rectangular form and polar form of coordinates. Then we have to explain how the polar and the rectangular form can be found with the help of a simple example for a complex equation.
Complete step by step solution:
Polar form :
The polar form of a complex number is nothing but the way to represent a complex number apart from the rectangular form. The polar form is a form of complex number which is denoted by the length and the angle of its vector . But in the polar form, we used to represent the complex numbers as the combination of modulus and argument. The polar coordinates are
Now we can find the polar coordinate form of the complex number.
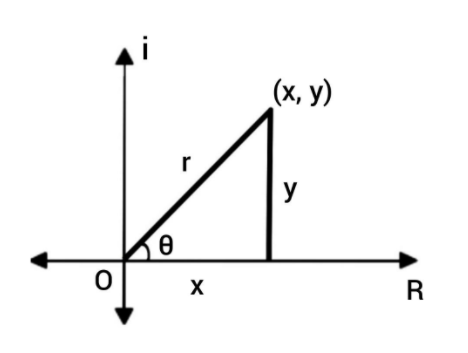
The x axis is the real axis and the y axis is the imaginary axis. We have found the real and complex components in terms of
From the Pythagoras theorem,
By using the trigonometric ratios, we know that cosine is adjacent to the Hypotenuse.
On multiplying both sides by
We get,
Also sine is opposite to the hypotenuse.
On multiplying both sides by
We get,
The rectangular form of a complex number is given by
On substituting
We get,
By taking
We get,
Where
Thus the polar form of the complex number
Rectangular form :
Rectangular form of a complex number is denoted by its respective horizontal and vertical components.
The rectangular coordinate form of a complex number is
The rectangular coordinates are
Now we consider a complex number
First we can find the polar form of
The general form of the complex number is
By comparing,
We get,
First we can find
On substituting the values,
We get,
On simplifying,
We get,
Also we know that
Thus by substituting the values,
We get,
Now we need to find
We know that
On substituting
We get,
On simplifying,
We get
Thus the polar form of
The polar coordinates are
We need to know that
The polar form of a complex number is nothing but another way to represent a complex number apart from the rectangular form
Note:
First we should know what a complex number is. Mathematically, complex numbers are represented as
Complete step by step solution:
Polar form :
The polar form of a complex number is nothing but the way to represent a complex number apart from the rectangular form. The polar form is a form of complex number which is denoted by the length and the angle of its vector . But in the polar form, we used to represent the complex numbers as the combination of modulus and argument. The polar coordinates are
Now we can find the polar coordinate form of the complex number.

The x axis is the real axis and the y axis is the imaginary axis. We have found the real and complex components in terms of
From the Pythagoras theorem,
By using the trigonometric ratios, we know that cosine is adjacent to the Hypotenuse.
On multiplying both sides by
We get,
Also sine is opposite to the hypotenuse.
On multiplying both sides by
We get,
The rectangular form of a complex number is given by
On substituting
We get,
By taking
We get,
Where
Thus the polar form of the complex number
Rectangular form :
Rectangular form of a complex number is denoted by its respective horizontal and vertical components.
The rectangular coordinate form of a complex number is
The rectangular coordinates are
Now we consider a complex number
First we can find the polar form of
The general form of the complex number is
By comparing,
We get,
First we can find
On substituting the values,
We get,
On simplifying,
We get,
Also we know that
Thus by substituting the values,
We get,
Now we need to find
We know that
On substituting
We get,
On simplifying,
We get
Thus the polar form of
The polar coordinates are
We need to know that
The polar form of a complex number is nothing but another way to represent a complex number apart from the rectangular form
Note:
First we should know what a complex number is. Mathematically, complex numbers are represented as
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




