Question

Let a and b be any two numbers satisfying \[\dfrac{1}{{{a^2}}} + \dfrac{1}{{{b^2}}} = \dfrac{1}{4}\] . Then, the foot of perpendicular from the origin on the variable line, \[\dfrac{x}{a} + \dfrac{y}{b} = 1\] lies on:
A) A hyperbola with each semi axis \[ = \sqrt 2 \]
B) A hyperbola with each semi axis \[ = 2\]
C) A circle of radius \[ = 2\]
D) A circle of radius \[ = \sqrt 2 \]

Answer 1

Hint: Here we will first find the slope of the perpendicular line with the help of the given line and then find the equation of the perpendicular line using the given points and then finally use the given condition to get the answer.

Complete step-by-step answer:
                    

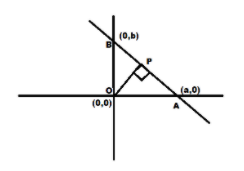
The given equation of the line AB is:-
\[\dfrac{x}{a} + \dfrac{y}{b} = 1\]
Simplifying it by taking LCM we get:-
\[
  \dfrac{{b\left( x \right) + a\left( y \right)}}{{ab}} = 1 \\
   \Rightarrow bx + ay = ab \\
 \]
Now writing it in the standard slope point form of equation i.e. \[y = mx + c\] we get:-
\[ay = - bx + ab\]
Dividing whole equation by a we get:-
\[
  y = \dfrac{{ - bx + ab}}{a} \\
   \Rightarrow y = \dfrac{{ - b}}{a}x + b....................................\left( 1 \right) \\
 \]
Now comparing it with standard form i.e. \[y = mx + c\] we get:-
\[m = \dfrac{{ - b}}{a}\]
Now let the slope of the perpendicular line be \[{m_p}\]
Then we know that if two lines are perpendicular then the product of their slopes is equal to -1.
Now since the given line and the line with the slope \[{m_p}\] are perpendicular
Therefore,
\[m \times {m_p} = - 1\]
Putting the known values we get:-
\[\dfrac{{ - b}}{a} \times {m_p} = - 1\]
Now solving for \[{m_p}\] we get:-
\[
  \left( { - b} \right){m_p} = \left( { - 1} \right)\left( a \right) \\
   \Rightarrow {m_p} = \dfrac{{ - a}}{{ - b}} \\
   \Rightarrow {m_p} = \dfrac{a}{b} \\
 \]
Now we will form the equation of the perpendicular line OP using slope point form.
The slope point form of a line with slope m and passing through point \[\left( {{x_1},{y_1}} \right)\] is given by:-
\[y - {y_1} = m\left( {x - {x_1}} \right)\]
Now since it is given that the perpendicular line OP passes through origin (0,0) and also its slope is \[{m_p} = \dfrac{a}{b}\]
Therefore, the equation of OP is:-
\[y - 0 = {m_p}\left( {x - 0} \right)\]
Putting in the value of \[{m_p}\] we get:-
\[
  y - 0 = \dfrac{a}{b}\left( {x - 0} \right) \\
   \Rightarrow y = \dfrac{a}{b}x...................\left( 2 \right) \\
 \]
Now in order to find the foot of perpendicular of OP on AB we need to find their point of intersection P.
Hence we will solve the equations 1 and 2 to find the values of x and y.
Therefore, putting the value of y from equation 2 in equation 1 we get:-
\[\dfrac{a}{b}x = \dfrac{{ - b}}{a}x + b\]
Now solving for x we get:-
\[\dfrac{a}{b}x + \dfrac{b}{a}x = b\]
Now taking LCM we get:-
\[
  \dfrac{{{a^2}x + {b^2}x}}{{ab}} = b \\
  \dfrac{{x\left( {{a^2} + {b^2}} \right)}}{{ab}} = b \\
 \]
Now cross-multiplying we get:-
\[
  x\left( {{a^2} + {b^2}} \right) = a{b^2} \\
   \Rightarrow x = \dfrac{{a{b^2}}}{{{a^2} + {b^2}}}................................\left( 3 \right) \\
 \]
Now putting this value in equation 2 we get:-
\[y = \dfrac{a}{b}\left( {\dfrac{{a{b^2}}}{{{a^2} + {b^2}}}} \right)\]
Simplifying it further we get:-
\[y = \dfrac{{{a^2}b}}{{{a^2} + {b^2}}}..........................\left( 4 \right)\]
Now squaring and adding equations 3 and 4 we get:-
\[{x^2} + {y^2} = {\left( {\dfrac{{a{b^2}}}{{{a^2} + {b^2}}}} \right)^2} + {\left( {\dfrac{{{a^2}b}}{{{a^2} + {b^2}}}} \right)^2}\]
Solving it further we get:-
\[
  {x^2} + {y^2} = \dfrac{{{a^2}{b^4}}}{{{{\left( {{a^2} + {b^2}} \right)}^2}}} + \dfrac{{{a^4}{b^2}}}{{{{\left( {{a^2} + {b^2}} \right)}^2}}} \\
   \Rightarrow {x^2} + {y^2} = \dfrac{{{a^2}{b^4} + {a^4}{b^2}}}{{{{\left( {{a^2} + {b^2}} \right)}^2}}} \\
 \]
Taking \[{a^2}{b^2}\] common we get:-
\[
  {x^2} + {y^2} = \dfrac{{{a^2}{b^2}\left( {{b^2} + {a^2}} \right)}}{{{{\left( {{a^2} + {b^2}} \right)}^2}}} \\
   \Rightarrow {x^2} + {y^2} = \dfrac{{{a^2}{b^2}}}{{{a^2} + {b^2}}}........................\left( 5 \right) \\
 \]
Now it is given that:-
\[\dfrac{1}{{{a^2}}} + \dfrac{1}{{{b^2}}} = \dfrac{1}{4}\]
Simplifying it and taking LCM we get:-
\[\dfrac{{{b^2} + {a^2}}}{{{a^2}{b^2}}} = \dfrac{1}{4}\]
Now taking reciprocal we get:-
\[\dfrac{{{a^2}{b^2}}}{{{a^2} + {b^2}}} = 4\]
Putting this value in equation 5 we get:-
\[{x^2} + {y^2} = 4\]
which is equation of a circle with radius \[ = 2\]

So, the correct answer is “Option C”.

Note: In such questions we have to make use of the given information and then find the desired result. Students should take a note that if two lines are perpendicular then the product of their slopes is equal to -1. Also, the equation of a circle with center at (0,0) and radius r is given by:-
\[{x^2} + {y^2} = {r^2}\]