
How do you know which method to use to solve triangles?
Answer
476.1k+ views
Hint: To solve a triangle means, finding the length of its sides and measure of its angle. We can solve the triangle by using either Law of Sines which states that
Complete step by step answer:
To solve a triangle, we can use the Sine rule or Cosine rule. It depends on the given components of the triangle that is the number of sides or number of angles we know, which rule should be used.
We will now see the condition for which the Sine rule should be used to solve a triangle, see the following figure,
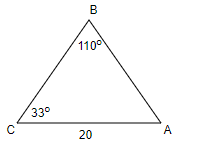
As we can see from the above
Now, from the figure we have the measure of
We will see the condition for which the Cosine rule should be used to solve a triangle, see the figure given below,
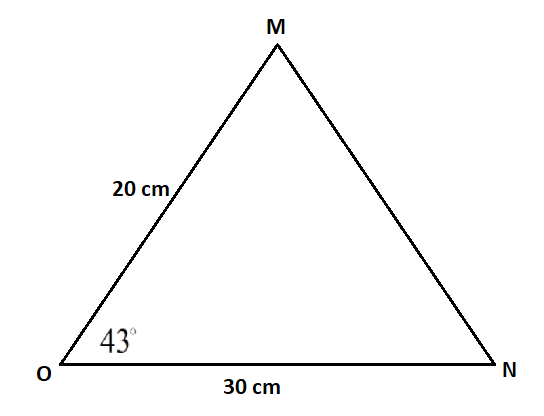
From the above figure, we can say that
Note:
There can be other conditions also where a particular rule should be used other than these two. If one of the angles is a right angle then the Cosine rule becomes
Complete step by step answer:
To solve a triangle, we can use the Sine rule or Cosine rule. It depends on the given components of the triangle that is the number of sides or number of angles we know, which rule should be used.
We will now see the condition for which the Sine rule should be used to solve a triangle, see the following figure,

As we can see from the above
Now, from the figure we have the measure of
We will see the condition for which the Cosine rule should be used to solve a triangle, see the figure given below,

From the above figure, we can say that
Note:
There can be other conditions also where a particular rule should be used other than these two. If one of the angles is a right angle then the Cosine rule becomes
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




