
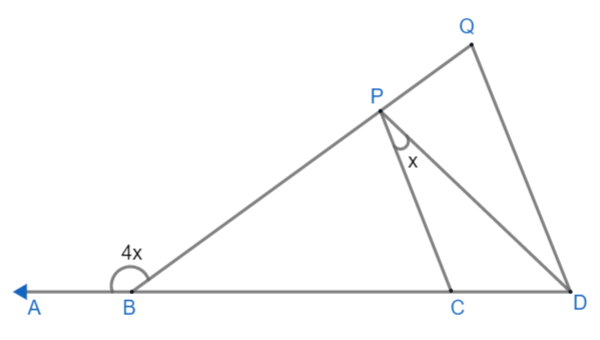
In this given figure, ABCD and BPQ line. BP = BC and DQ || CP. Prove that
(I) CP = CD
(II) DP bisects angle CDQ.

Answer
495.6k+ views
Hint: In this question, we are given BP = BC and DQ || CP. Also,
(I) Exterior angle product: Exterior angle of a triangle is equal to the sum of the interior opposite angles.
(II) Isosceles triangle property: Angles corresponding to equal sides is an isosceles triangle and are always equal.
(III) Converse of isosceles triangle property: If two angles are equal in a triangle, then the corresponding side of equal angles are also equal.
(IV) If two lines are parallel and a transversal forms corresponding angles with parallel lines then corresponding angles are equal.
Complete step-by-step solution
Here we are given the diagram as:

Here BP = BC,
(i) Firstly, we need to prove CP = CD.
As we can see from triangle BPC that BP = BC, therefore
Now as we can see
So using exterior angle property, which states that, an exterior angle is equal to the sum of two opposite interior angles, we get:
From (1),
Also,
Hence
Now using exterior angle property in the
As we know,
Hence in the triangle
As we know, from the converse of the isosceles triangle property, sides corresponding to equal angles are also equal. Therefore, CP = CD. Hence proved.
(ii) Now let us prove DP bisects
Hence we need to prove
From the diagram, we know that CP || DQ.
Since CP and DQ are parallel lines and CD acts as transversal, so
As equivalent earlier,
Now, as we can see,
Also we know
Now
Note: Students should know all the properties of triangles before solving these sums. While using exterior angle property, make sure to take the sum of opposite interior angles only. We can take
(I) Exterior angle product: Exterior angle of a triangle is equal to the sum of the interior opposite angles.
(II) Isosceles triangle property: Angles corresponding to equal sides is an isosceles triangle and are always equal.
(III) Converse of isosceles triangle property: If two angles are equal in a triangle, then the corresponding side of equal angles are also equal.
(IV) If two lines are parallel and a transversal forms corresponding angles with parallel lines then corresponding angles are equal.
Complete step-by-step solution
Here we are given the diagram as:

Here BP = BC,
(i) Firstly, we need to prove CP = CD.
As we can see from triangle BPC that BP = BC, therefore
Now as we can see
So using exterior angle property, which states that, an exterior angle is equal to the sum of two opposite interior angles, we get:
From (1),
Also,
Hence
Now using exterior angle property in the
As we know,
Hence in the triangle
As we know, from the converse of the isosceles triangle property, sides corresponding to equal angles are also equal. Therefore, CP = CD. Hence proved.
(ii) Now let us prove DP bisects
Hence we need to prove
From the diagram, we know that CP || DQ.
Since CP and DQ are parallel lines and CD acts as transversal, so
As equivalent earlier,
Now, as we can see,
Also we know
Now
Note: Students should know all the properties of triangles before solving these sums. While using exterior angle property, make sure to take the sum of opposite interior angles only. We can take
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




