
In the pulley system shown, if radii of the bigger and smaller pulley are
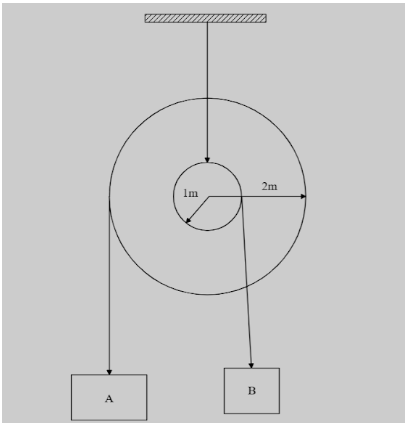

Answer
486k+ views
2 likes
Hint: Since the bodies will be acting with gravity on both sides of the pulleys, the pulleys are going to experience angular acceleration. Since the bodies are connected to each other, the angular acceleration of the bodies is going to be equal.
Formulas used: Since the pulley appears to be going through a circular motion from the origin, we will use the formula
Complete Answer:
We know that when a body is suspended by a pulley it experiences a tension that opposes gravity and the gravity itself acting on it. But considering two such pulleys connected to each other, both the pulleys will be undergoing angular acceleration.
The angular acceleration that both the bodies undergo will be given by the formula,
For body A,
For body B,
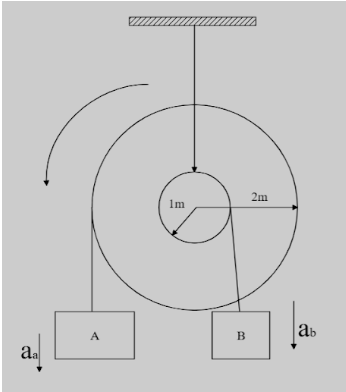
But as we can infer from the diagram that the pulleys are connected to each other and thus the rotation of the pulleys and by them the bodies A and B are limited. And thus, the angular acceleration of the bodies is going to equal. That is,
We know from the problem,
Thus, the acceleration of the block B will be
Hence the correct answer will be option D.
Note:
The formula
Formulas used: Since the pulley appears to be going through a circular motion from the origin, we will use the formula
Complete Answer:
We know that when a body is suspended by a pulley it experiences a tension that opposes gravity and the gravity itself acting on it. But considering two such pulleys connected to each other, both the pulleys will be undergoing angular acceleration.
The angular acceleration that both the bodies undergo will be given by the formula,
For body A,
For body B,

But as we can infer from the diagram that the pulleys are connected to each other and thus the rotation of the pulleys and by them the bodies A and B are limited. And thus, the angular acceleration of the bodies is going to equal. That is,
We know from the problem,
Thus, the acceleration of the block B will be
Hence the correct answer will be option D.
Note:
The formula
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




