Question

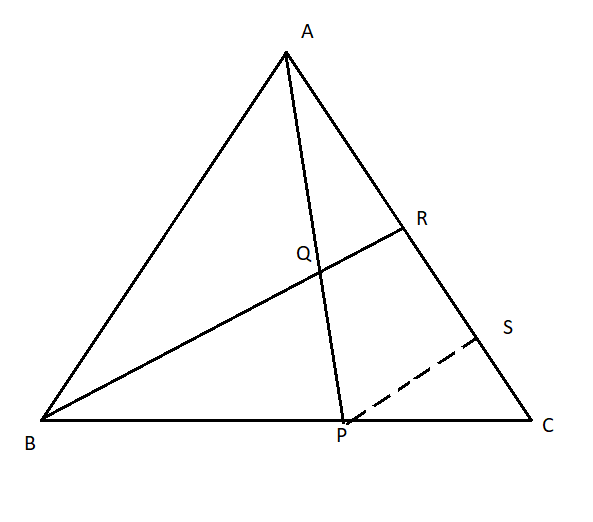
In the given figure, P is the midpoint of BC and Q is the midpoint of AP. If BQ when produced meets AC at R. Prove that ${\text{RA = }}\dfrac{1}{3}{\text{CA}}$.

Answer 1

Hint: In this to prove the given result we have to do some construction. Initially, we have to draw PS parallel to the BR. Then, using properties of midpoint of lines in the triangle we have to find different relations of AR, RS, SC.

Complete step by step answer:
Given: A triangle ABC in which P is the midpoint of BC, Q is the midpoint of AP, such that when BQ is produced it meets AC at R.
Construction: Draw a line PS in such a way that PS||BR, meeting AC at S.

In $\Delta $BCR, P is the mid-point of BC and PS||BR. Then S is the mid-point of CR.
$ \Rightarrow {\text{CS}} = {\text{SR }}$ eq.1
In $\Delta $APS, Q is the mid-point of AP and QR||PS. Then R is the mid-point of AS.
$ \Rightarrow {\text{RA}} = {\text{SR }}$ eq.2
From eq.1 and eq.2, we get
$ \Rightarrow {\text{RA = SR = CS}}$ eq.3
We can write CA as sum of CS, SR, RA
$
   \Rightarrow {\text{ CA = CS + SR + RA}} \\
   \Rightarrow {\text{ CA = }}3{\text{RA }} \\
   \Rightarrow {\text{ AR = }}\dfrac{1}{3}{\text{CA }} \\
$
  ( from eq.1 , eq.2 and eq.3)
Hence proved.

Note:
Whenever you get this type of problem the key concept is to make a rough diagram and observe what changes you should have to make so that question is solvable. And you have to learn the basic properties of triangle and line.