
In the cube of side '
(A)
(B)
(C)
(D)

Answer
484.8k+ views
1 likes
Hint: In geometry, a position or position vector, also known as location vector or radius vector, is a Euclidean vector that represents the position of a point P in space in relation to an arbitrary reference origin O. Calculate the coordinates of the mid-points of the faces and compute them in the formula for position vector.
Formula Used: The formulae used in the solution are given here.
Position vector
Where,
Complete Answer:
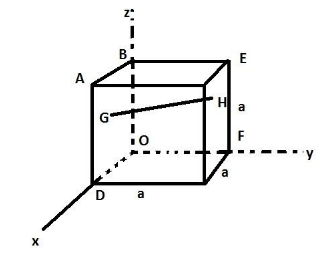
It has been given that the length of the sides of the cube is
The centre of face ABOD is represented by point G as shown in the figure provided in the question. Similarly, the point H is the centre of the face BEFO of the cube. The line segment joining the two points on the opposite faces has been shown as the GH line.
The position vector is used to specify the position of a certain body. Knowing the position of a body is vital when it comes to describing the motion of that body. The position vector of an object is measured from the origin, in general.
Position vector
Where,
In the given figure, the coordinate of point O is (0,0,0), point D is (a,0,0), and B is(0,0,a). The coordinates of point G is therefore,
The coordinates of point F is (0,a,0) and thus, the coordinates of point H is
The value of the required vector is given by,
The vector
The vector
Assigning the respective values in the equation, we get,
Rearranging the values we get,
Hence the correct answer is Option B.
Note:
In three dimensions, any set of three-dimensional coordinates and their corresponding basis vectors can be used to define the location of a point in space—whichever is the simplest for the task at hand may be used. The position in a three-dimensional space is defined with respect to the coordinates of the points joining the two.
Formula Used: The formulae used in the solution are given here.
Position vector
Where,
Complete Answer:
It has been given that the length of the sides of the cube is
The centre of face ABOD is represented by point G as shown in the figure provided in the question. Similarly, the point H is the centre of the face BEFO of the cube. The line segment joining the two points on the opposite faces has been shown as the GH line.
The position vector is used to specify the position of a certain body. Knowing the position of a body is vital when it comes to describing the motion of that body. The position vector of an object is measured from the origin, in general.
Position vector
Where,
In the given figure, the coordinate of point O is (0,0,0), point D is (a,0,0), and B is(0,0,a). The coordinates of point G is therefore,
The coordinates of point F is (0,a,0) and thus, the coordinates of point H is
The value of the required vector is given by,
The vector
The vector
Assigning the respective values in the equation, we get,
Rearranging the values we get,
Hence the correct answer is Option B.
Note:
In three dimensions, any set of three-dimensional coordinates and their corresponding basis vectors can be used to define the location of a point in space—whichever is the simplest for the task at hand may be used. The position in a three-dimensional space is defined with respect to the coordinates of the points joining the two.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

How do I convert ms to kmh Give an example class 11 physics CBSE

Where can free central placentation be seen class 11 biology CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE




