
In the circuit shown below, the reading of the voltmeter V is
A.12V
B.8V
C.20V
D.16V
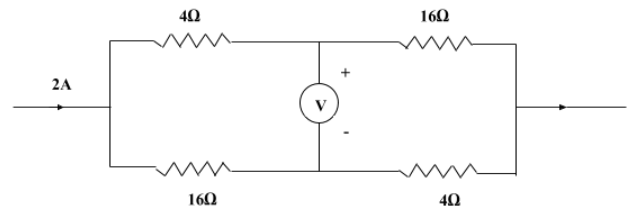

Answer
504k+ views
1 likes
Hint: As we can see, the two resistors which are connected to the voltmeter are connected in parallel. So, first find the potential difference along the path where the
Complete answer:
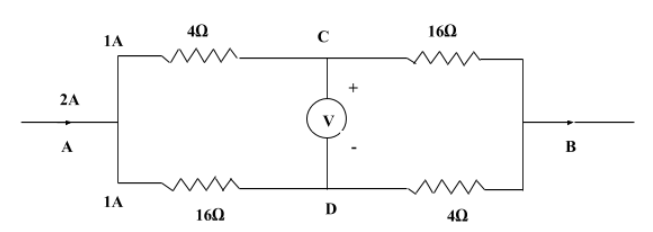
Let the potential at point A be
Potential difference across points A and C is given by,
From Ohm’s law we know,
Therefore, potential across
Substituting this value in the equation. (1) we get,
Similarly, potential difference across points A and D is given by,
Potential across
Substituting this value in the equation. (1) we get,
Thus, potential difference across points C and D is given by,
Substituting equation. (2) and (4) in above equation we get,
Hence, the reading of the voltmeter V is 12V.
So, the correct answer is option A i.e. 12V.
Note:
When the resistors are connected in series, there is only one path for the current to flow. Thus, equal current flows through each resistor. But, the potential drop across all the resistors in series may not be the same.
When the resistors are connected in parallel, there are many paths for the current to flow. The current may not be the same through each path in the circuit. But, the potential drop across all the resistors connected in parallel is the same.
Complete answer:

Let the potential at point A be
Potential difference across points A and C is given by,
From Ohm’s law we know,
Therefore, potential across
Substituting this value in the equation. (1) we get,
Similarly, potential difference across points A and D is given by,
Potential across
Substituting this value in the equation. (1) we get,
Thus, potential difference across points C and D is given by,
Substituting equation. (2) and (4) in above equation we get,
Hence, the reading of the voltmeter V is 12V.
So, the correct answer is option A i.e. 12V.
Note:
When the resistors are connected in series, there is only one path for the current to flow. Thus, equal current flows through each resistor. But, the potential drop across all the resistors in series may not be the same.
When the resistors are connected in parallel, there are many paths for the current to flow. The current may not be the same through each path in the circuit. But, the potential drop across all the resistors connected in parallel is the same.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




