Question

In an equilateral triangle prove that the centroid and centre of the circumcircle coincide.

Answer 1

Hint: In this question, first of all we will draw an equilateral triangle inscribed in a circle and also draw the three medians of the triangle. To show that G is the centre of the circumcircle, we will show that G is equidistant from A, B and C.

Complete step by step solution:
Let us first make diagram:

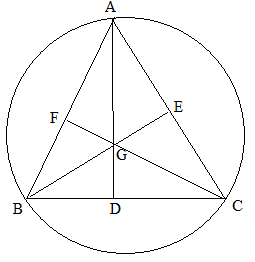
Construction: We have drawn median AD, BE and CF.
Let G be the centroid of triangle ABC.
Triangle ABC is an equilateral triangle.
$\therefore $ AB = BC = CA. And $\angle ABC = \angle BAC = \angle BCA = {60^0}$
And we can also say that $\dfrac{{AB}}{2} = \dfrac{{BC}}{2} = \dfrac{{CA}}{2}$ $ \Rightarrow $ BF =AF= BD = EC=AE.
Now, in $\vartriangle BFC$and$\vartriangle BEC$, we have:
BC= BC (Common Side)
$\angle FBC = \angle ECB = {60^0}$
BF = EC.

So, by SAS rule of congruence, we can write:
$\vartriangle BFC$ $ \cong $$\vartriangle BEC$.
$\because $ The two triangles are congruent. So, all angles and sides of one triangle are equal to corresponding angles and sides of another triangle.
Therefore , we have BE= CF. (1)
Now, in $\vartriangle ABE$and$\vartriangle ABD$, we have:
AB= AB (Common Side)
$\angle BAE = \angle ABD = {60^0}$
BD = AE.

So, by SAS rule of congruence, we can write:
$\vartriangle ABE$$ \cong $$\vartriangle ABD$
$\because $ The two triangles are congruent. So, all angles and sides of one triangle are equal to corresponding angles and sides of another triangle.
Therefore , we have BE= AD. (2)
From equation 1 and 2, we get:
AD = BE = CF. (3)
Now , we know that the G( the centroid) of the triangle divides the median in a 2:3 ratio.
So, on dividing the equation 4 by 2/3, we get:
$\dfrac{2}{3}AD = \dfrac{2}{3}BE = \dfrac{2}{3}CF.$
GA = GB = GC.
So, we can say that G is equidistant from the three vertices A. B and C.
Hence, it is proved that G is centroid as well as centre of circumcircle of the equilateral triangle.

Note: In this question, you should know the definition of centroid and centre of circumcircle of a triangle. Centroid is the intersection of all the three medians of the triangle and the centre of the circumcircle is the point of intersection of all the three perpendicular bisectors drawn from the vertex to the opposite side.