
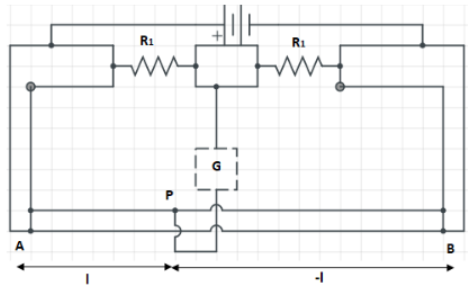
In a meter bridge, the wire of the length 1m has a non-uniform cross- section such that, the variation

Answer
505.8k+ views
2 likes
Hint: According to wheat stone’s bridge when the galvanometer shows zero deflection, we can use balanced condition. Therefore use balanced conditions. Then take resistances of point AD and DB as
Complete answer:
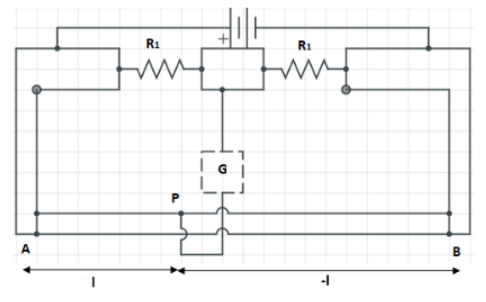
Question stated that
Total length of wire AB is 1m which has non- uniform cross-section. Thus, for the given wire:
Where, C = constant
It is mentioned that the resistance attached is the same. Let
Now use equation (1)
Put
Integrate, we get
Similarly for
Therefore,
According to condition given in equation (2), we get,
Hence the length of AD is 0.25m.
Therefore option (C) is the correct option.
Note:
Meter Bridge is the modification of the wheat stone’s network. Wheatstone’s network is used to determine the value of the unknown resistance. Since the length of the wire used is one meter therefore it is called a meter Bridge. The balancing condition for Wheatstone’s network can also be determined by using ohm’s law.
Complete answer:

Question stated that
Total length of wire AB is 1m which has non- uniform cross-section. Thus, for the given wire:
Where, C = constant
It is mentioned that the resistance attached is the same. Let
Now use equation (1)
Put
Integrate, we get
Similarly for
Therefore,
According to condition given in equation (2), we get,
Hence the length of AD is 0.25m.
Therefore option (C) is the correct option.
Note:
Meter Bridge is the modification of the wheat stone’s network. Wheatstone’s network is used to determine the value of the unknown resistance. Since the length of the wire used is one meter therefore it is called a meter Bridge. The balancing condition for Wheatstone’s network can also be determined by using ohm’s law.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




