
If the larger base of an isosceles trapezoid equals a diagonal and the smaller base equals the altitude, then the ratio of smaller base to the larger base is:
(a)
(b)
(c)
(d)
(e)
Answer
510.6k+ views
1 likes
Hint: First of all draw the figure according to the information given in the above problem. As the trapezoid given is the isosceles trapezoid meaning its lower base angles are congruent along with the upper base angles too. And both the diagonals of the trapezoid are also congruent. Let us assume the smaller base of the diagonal as “s” and the longer base as “l”. Then length of the diagonals become “l” and altitudes of the trapezoid become “s” because it is given that the larger base of an isosceles trapezoid equals a diagonal and the smaller base equals the altitude. After that by using the Pythagoras theorem and the basic geometry we can find the ratio of “s” and “l”.
Complete step by step answer:
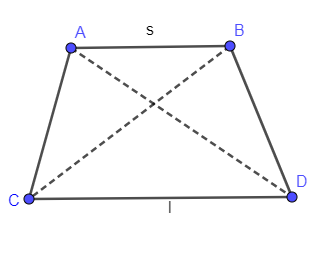
In the below diagram, we have shown an isosceles trapezoid ABCD with larger and smaller bases as “l” and “s” and the two diagonals AD and BC.
Now, we are going to draw the two altitudes AE and BF on the base CD of the trapezoid.
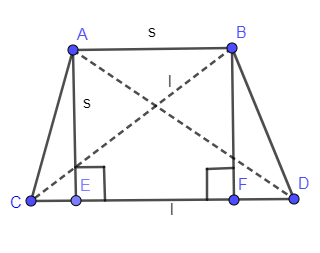
Let us take two triangles AEC and BFD and we are going to prove the congruence of these two triangles.
In
It is the property of the isosceles trapezoid that altitudes are equal.
The above angles are equal because the altitudes are making an angle of
The above angles are equal because there is a property of the isosceles trapezoid that the lower base angles are equal.
Hence, by AAS congruence
We know that if two triangles are congruent then by CPCT (Corresponding part of the congruent triangles) sides CE and DF are equal.
As we have proved above that:
Let us assume that lengths of CE and DF are equal to x.
So, the summation of CE, EF and FD is equal to “l”.
The length of the side EF is “s” from the figure.
Adding CE, EF and FD and equating them to “l” we get,
We are again drawing the diagram that we have drawn above for the better understanding of the solution.
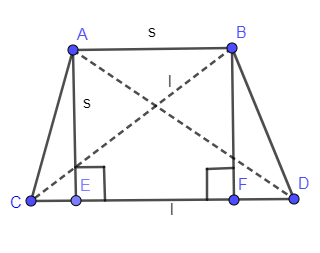
Now, we are taking the right angled triangle AED.
Now, applying Pythagoras theorem in this right angled triangle we get,
Substituting hypotenuse as “l”, perpendicular as “s” and base as
Rewriting the above equation we get,
Taking
Solving the above equation by equating each bracket to 0 we get,
As “l” and “s” are representing the sides of the trapezoid which cannot be negative so eq. (1) is rejected and the solution we are getting is equal to:
Hence, we got the ratio of a smaller to larger base is equal to
So, the correct answer is “Option D”.
Note: The problem demands the knowledge of the properties of isosceles trapezoid and Pythagoras theorem if you miss any of the properties of isosceles trapezoid then you get stuck in this problem.
And always check the solutions that you are getting from the quadratic equation whether those solutions are satisfying the conditions of the problem or not. For e.g. in this problem, after solving the following quadratic equation:
We are getting two solutions:
We have rejected the first solution because the sides of the trapezoid cannot be negative.
Complete step by step answer:
In the below diagram, we have shown an isosceles trapezoid ABCD with larger and smaller bases as “l” and “s” and the two diagonals AD and BC.

Now, we are going to draw the two altitudes AE and BF on the base CD of the trapezoid.

Let us take two triangles AEC and BFD and we are going to prove the congruence of these two triangles.
In
It is the property of the isosceles trapezoid that altitudes are equal.
The above angles are equal because the altitudes are making an angle of
The above angles are equal because there is a property of the isosceles trapezoid that the lower base angles are equal.
Hence, by AAS congruence
We know that if two triangles are congruent then by CPCT (Corresponding part of the congruent triangles) sides CE and DF are equal.
As we have proved above that:
Let us assume that lengths of CE and DF are equal to x.
So, the summation of CE, EF and FD is equal to “l”.
The length of the side EF is “s” from the figure.
Adding CE, EF and FD and equating them to “l” we get,
We are again drawing the diagram that we have drawn above for the better understanding of the solution.

Now, we are taking the right angled triangle AED.
Now, applying Pythagoras theorem in this right angled triangle we get,
Substituting hypotenuse as “l”, perpendicular as “s” and base as
Rewriting the above equation we get,
Taking
Solving the above equation by equating each bracket to 0 we get,
As “l” and “s” are representing the sides of the trapezoid which cannot be negative so eq. (1) is rejected and the solution we are getting is equal to:
Hence, we got the ratio of a smaller to larger base is equal to
So, the correct answer is “Option D”.
Note: The problem demands the knowledge of the properties of isosceles trapezoid and Pythagoras theorem if you miss any of the properties of isosceles trapezoid then you get stuck in this problem.
And always check the solutions that you are getting from the quadratic equation whether those solutions are satisfying the conditions of the problem or not. For e.g. in this problem, after solving the following quadratic equation:
We are getting two solutions:
We have rejected the first solution because the sides of the trapezoid cannot be negative.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Write examples of herbivores carnivores and omnivo class 10 biology CBSE

Difference between mass and weight class 10 physics CBSE

List out three methods of soil conservation

Leap year has days A 365 B 366 C 367 D 368 class 10 maths CBSE

How does Tommy describe the old kind of school class 10 english CBSE




