
If the angles of the depression and elevation of the top of a tower of height h from the top and bottom of a second tower are x and y respectively then the height of the second tower is:
(a) h (cot y + cot x)
(b) h (tan x + tan y)
(c) h (1 + tan x cot y)
(d) h (tan y cot x + 1)
Answer
528.6k+ views
Hint:First of all, draw a figure in which there are two towers AB and CD of height h and
Complete step-by-step answer:
In this question, we are given that the angles of depression and elevation of the top of a tower of height h from the top and bottom of a second tower are x and y respectively then we have to find the height of the second tower. Let us assume two towers of height h and
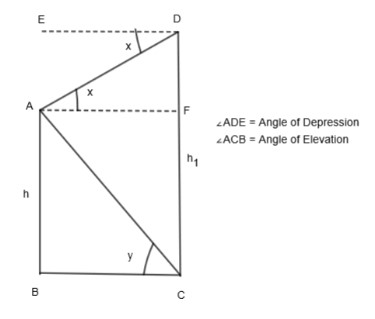
We know that DE is parallel to FA and
We know that,
Let us consider a triangle ABC, so we get,
By substituting the value of
Let us consider triangle AFD, we get,
By substituting the values of
We can write DF as DC – FC. We know that FC = AB = h and
By substituting the value of AF in equation (ii), we get,
Now, by equating the value of BC from equation (i) and (iii), we get,
By canceling the like terms and using
So, we get the height of the second tower as h (1 + tan x cot y).
Hence, the option (c) is the right answer.
Note: In this type of questions, the most important point is to make the proper diagram to visualize the question. In this question, many students make this mistake of making the angle of depression and elevation from the top of tower 1 to the top and bottom of tower 2 by taking
Complete step-by-step answer:
In this question, we are given that the angles of depression and elevation of the top of a tower of height h from the top and bottom of a second tower are x and y respectively then we have to find the height of the second tower. Let us assume two towers of height h and

We know that DE is parallel to FA and
We know that,
Let us consider a triangle ABC, so we get,
By substituting the value of
Let us consider triangle AFD, we get,
By substituting the values of
We can write DF as DC – FC. We know that FC = AB = h and
By substituting the value of AF in equation (ii), we get,
Now, by equating the value of BC from equation (i) and (iii), we get,
By canceling the like terms and using
So, we get the height of the second tower as h (1 + tan x cot y).
Hence, the option (c) is the right answer.
Note: In this type of questions, the most important point is to make the proper diagram to visualize the question. In this question, many students make this mistake of making the angle of depression and elevation from the top of tower 1 to the top and bottom of tower 2 by taking
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




