
If ABCD is a regular tetrahedron with the length of edge as
(1) Volume of the tetrahedron is
(2) Volume of the tetrahedron is
(3) The minimum distance of any vertex from the opposite face is
(4) The minimum distance of any vertex from the opposite face is
Answer
425.4k+ views
1 likes
Hint: This question is based on the basic concepts of triangles. The formula required for solving this question is that the area of the triangle is equal to,
Also, knowing the basics of the equilateral triangle would help, like the area of the triangle is
Complete step-by-step answer:
Now, we have a regular tetrahedron, that means we have all the sides equal.
Thus, the base of the tetrahedron is nothing but an equilateral triangle. Now, for calculating the volume of the figure we need to calculate
Now, let’s calculate the base area,
Base area =
We know that ABC is an equilateral triangle.
Substituting the value of
Now, we need to calculate the height and for that let’s draw a perpendicular from the other vertex to the base triangle.
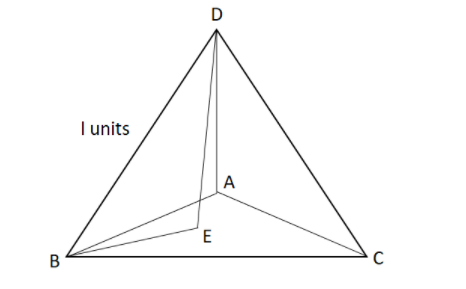
As the figure is symmetric, so the foot of the perpendicular coincides with the centroid of the base triangle as shown in the figure.
The perpendicular DE is drawn to the base of triangle ABC. Let’s join B to E and observe the triangle DBE.
DB =
The length of the Median is
Therefore, the length of BE =
Now, let’s calculate the value of the perpendicular DE, by using the Pythagoras theorem,
Therefore,
Substituting the values of DB and EB, we get,
Simplifying the expression, we get,
Taking square root on both sides of the equation, we get,
DE or the length of the perpendicular is
Now, the volume
Cancelling the common factors in numerator and denominator, we get,
Note: The question can get tedious if the calculations and geometrical mistakes are not taken in account. Please be careful while drawing the figure, keep it neat and mark the angles and length of the sides correctly. Use simplification rules to ease the arithmetic operations. One should remember the formulae for volume of the tetrahedron.
Also, knowing the basics of the equilateral triangle would help, like the area of the triangle is
Complete step-by-step answer:
Now, we have a regular tetrahedron, that means we have all the sides equal.
Thus, the base of the tetrahedron is nothing but an equilateral triangle. Now, for calculating the volume of the figure we need to calculate
Now, let’s calculate the base area,
Base area =
We know that ABC is an equilateral triangle.
Substituting the value of
Now, we need to calculate the height and for that let’s draw a perpendicular from the other vertex to the base triangle.

As the figure is symmetric, so the foot of the perpendicular coincides with the centroid of the base triangle as shown in the figure.
The perpendicular DE is drawn to the base of triangle ABC. Let’s join B to E and observe the triangle DBE.
DB =
The length of the Median is
Therefore, the length of BE =
Now, let’s calculate the value of the perpendicular DE, by using the Pythagoras theorem,
Therefore,
Substituting the values of DB and EB, we get,
Simplifying the expression, we get,
Taking square root on both sides of the equation, we get,
DE or the length of the perpendicular is
Now, the volume
Cancelling the common factors in numerator and denominator, we get,
Note: The question can get tedious if the calculations and geometrical mistakes are not taken in account. Please be careful while drawing the figure, keep it neat and mark the angles and length of the sides correctly. Use simplification rules to ease the arithmetic operations. One should remember the formulae for volume of the tetrahedron.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




