
If
A.
B.
C.
D.

Answer
535.2k+ views
Hint: In this question we will use some basic properties of circles and Pythagoras theorem. Here we have to find the radius of a circle that is inscribed in a quarter circle of given radius. To solve this question we will apply Pythagoras theorem i.e.
Complete step-by-step answer:
Given that, the radius of the quarter circle,
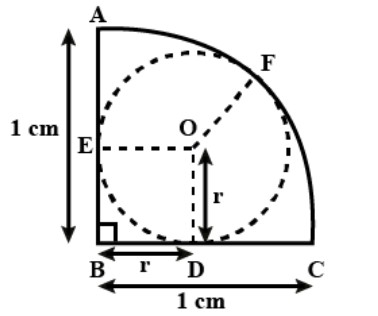
We know that a line joining the circumference to the centre of the circle is the radius of that circle.
So,
According to Pythagoras theorem,
Therefore,
Now, we have
We have ,
Then,
Now we will rationalise this value of r,
Here we get, r=
Hence, option (A) is correct.
Note: In this type of question first we will draw the proper figure as according to the statements given in the question. Then we will use the Pythagoras theorem to find out the radius of the inner circle . After that we will rationalise the radius that is obtained by the Pythagoras theorem and through this we will get the final required radius. Students should remember the formula of pythagoras and properties of the circle for solving these types of problems.
Complete step-by-step answer:
Given that, the radius of the quarter circle,

We know that a line joining the circumference to the centre of the circle is the radius of that circle.
So,
According to Pythagoras theorem,
Therefore,
Now, we have
We have ,
Then,
Now we will rationalise this value of r,
Here we get, r=
Hence, option (A) is correct.
Note: In this type of question first we will draw the proper figure as according to the statements given in the question. Then we will use the Pythagoras theorem to find out the radius of the inner circle . After that we will rationalise the radius that is obtained by the Pythagoras theorem and through this we will get the final required radius. Students should remember the formula of pythagoras and properties of the circle for solving these types of problems.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Write examples of herbivores carnivores and omnivo class 10 biology CBSE

Difference between mass and weight class 10 physics CBSE

List out three methods of soil conservation

Leap year has days A 365 B 366 C 367 D 368 class 10 maths CBSE

How does Tommy describe the old kind of school class 10 english CBSE




