
If a ball is thrown vertically upwards with speed u, the distance covered during the last t seconds of its ascent is:
A. ut
B.
C.
D. (u + gt)t
Answer
446.3k+ views
4 likes
Hint: When the ball will be thrown upwards it will undergo retardation due to gravity (g). In the last t seconds of its path during its ascent, it will start with some velocity v and reach a final velocity of zero before it starts to fall back (descent).
Formula used:
The final velocity of a body undergoing acceleration a with initial velocity u, in time interval t is:
v = u + at .
The distance covered by the (same) body in time interval t is given by:
Complete answer:
We are given that the ball is thrown vertically upwards with an initial velocity of u. We break the trajectory of ascent into three different times.
1. At t= 0, velocity is u
2. At t = T, velocity reaches zero (Let the total time of ascent be T).
3. At t = T-t, let the velocity be some v.
We are to determine the distance traveled between the points where time is T-t and T ( as T - (T-t) = t s).
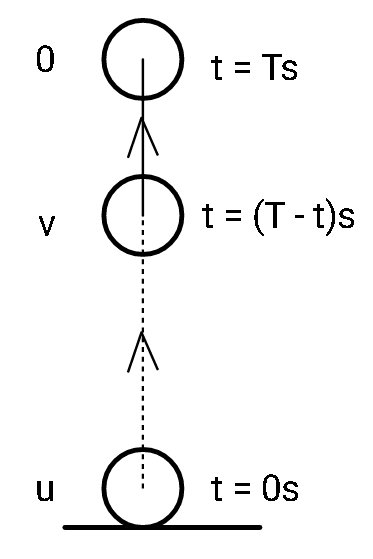
First, let us use the first law of motion to determine the velocity that the ball will attain after a time T-t s,
v = u + at
We know that a = -g due to retardation, so:
v = u - g(T-t).
For the second half of the journey, from T-t seconds to T seconds, we get
0 = v - gt
so, v= gt.
This will be the initial velocity for the ball when it enters the last t seconds of its ascent.
Therefore, we write in second law of motion:
In last t seconds, the ball will cover a distance of
Therefore, the correct answer is option (B).
Note:
Since the ball is undergoing retardation one should not forget to put a minus sign in front of g. Also one could get confused in the use of t. We always use a difference of final and initial time in the laws of motion. Here, in the first part of the ascent, the time interval was T-t - 0 = T-t seconds and in the second part it was T - (T-t) = t seconds.
Formula used:
The final velocity of a body undergoing acceleration a with initial velocity u, in time interval t is:
v = u + at .
The distance covered by the (same) body in time interval t is given by:
Complete answer:
We are given that the ball is thrown vertically upwards with an initial velocity of u. We break the trajectory of ascent into three different times.
1. At t= 0, velocity is u
2. At t = T, velocity reaches zero (Let the total time of ascent be T).
3. At t = T-t, let the velocity be some v.
We are to determine the distance traveled between the points where time is T-t and T ( as T - (T-t) = t s).

First, let us use the first law of motion to determine the velocity that the ball will attain after a time T-t s,
v = u + at
We know that a = -g due to retardation, so:
v = u - g(T-t).
For the second half of the journey, from T-t seconds to T seconds, we get
0 = v - gt
so, v= gt.
This will be the initial velocity for the ball when it enters the last t seconds of its ascent.
Therefore, we write in second law of motion:
In last t seconds, the ball will cover a distance of
Therefore, the correct answer is option (B).
Note:
Since the ball is undergoing retardation one should not forget to put a minus sign in front of g. Also one could get confused in the use of t. We always use a difference of final and initial time in the laws of motion. Here, in the first part of the ascent, the time interval was T-t - 0 = T-t seconds and in the second part it was T - (T-t) = t seconds.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

How do I convert ms to kmh Give an example class 11 physics CBSE

Where can free central placentation be seen class 11 biology CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE




