
How do you graph
Answer
478.2k+ views
Hint: We need to graph the given function. We will use the domain and some values of
Complete step-by-step solution:
The domain of the function
The graph of the cotangent function reaches arbitrarily large positive or negative values at these multiples of
Now, we will find some values of
Substituting
Substituting
Substituting
Substituting
The value of
Arranging the values of
The value of
Similarly, the value of
Now, we will use the points
Therefore, we get the graph
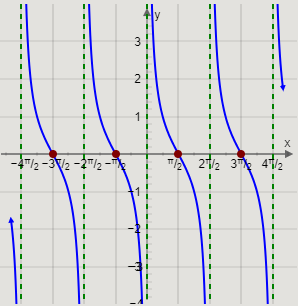
This is the required graph of the function
Note:
The period of the function
Complete step-by-step solution:
The domain of the function
The graph of the cotangent function reaches arbitrarily large positive or negative values at these multiples of
Now, we will find some values of
Substituting
Substituting
Substituting
Substituting
The value of
Arranging the values of
The value of
Similarly, the value of
Now, we will use the points
Therefore, we get the graph

This is the required graph of the function
Note:
The period of the function
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

How do I convert ms to kmh Give an example class 11 physics CBSE

Where can free central placentation be seen class 11 biology CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE




