
How do you graph
Answer
465.3k+ views
Hint: We have to draw the graph for the given function by taking different values of
Complete step-by-step solution:
Given graph is
The parent graph of the given graph
The domain of the function is when the bit inside the square root is greater than or equal to zero (otherwise it wouldn't be defined in terms of real numbers). We can find out when this is the case by solving the following inequality
Now subtracting 1 on both sides we get,
Now simplifying we get,
So required domain is equal to
Now The range of the parent graph,
Now to plot the graph we have to take any random values for
Now take
Now simplifying we get,
So again simplifying we get,
Now take
Now simplifying we get,
So again simplifying we get,
Now take
Now simplifying we get,
So again simplifying we get,
Now plotting the coordinates on the plane we get,
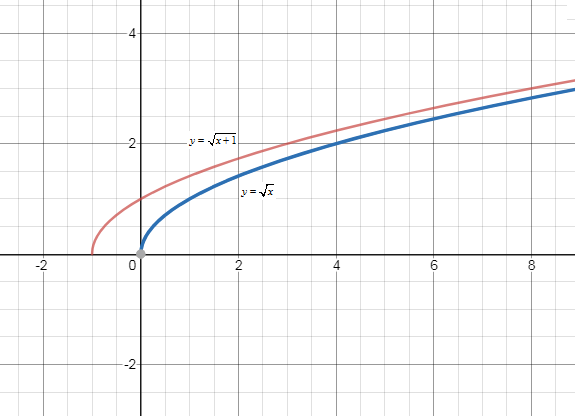
The parent graph of the given graph

and the domain for the given function is
Note: For plotting any type of graphs we should not take big values as it might get difficult to plot them on the graph, also from small graphs obtained will be the same as the graph obtained from the big values. And we should also know the concept of domain and range as the square root questions are only defined when
Complete step-by-step solution:
Given graph is
The parent graph of the given graph
The domain of the function is when the bit inside the square root is greater than or equal to zero (otherwise it wouldn't be defined in terms of real numbers). We can find out when this is the case by solving the following inequality
Now subtracting 1 on both sides we get,
Now simplifying we get,
So required domain is equal to
Now The range of the parent graph,
Now to plot the graph we have to take any random values for
Now take
Now simplifying we get,
So again simplifying we get,
Now take
Now simplifying we get,
So again simplifying we get,
Now take
Now simplifying we get,
So again simplifying we get,
Now plotting the coordinates on the plane we get,

The parent graph of the given graph

and the domain for the given function is
Note: For plotting any type of graphs we should not take big values as it might get difficult to plot them on the graph, also from small graphs obtained will be the same as the graph obtained from the big values. And we should also know the concept of domain and range as the square root questions are only defined when
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




