
From a disc of radius
A)
B)
C)
D)
Answer
514.5k+ views
1 likes
Hint: Moment of inertia of a disc is equal to half the product of mass of the disc and the square of radius of the disc. Parallel axis theorem states that the moment of inertia of a body about an axis parallel to the body passing through its centre is equal to the sum of moment of inertia of body about the axis passing through the centre and product of mass of the body times the square of distance between the two axes.
Complete step by step answer:
Moment of inertia of a body is a quantity which expresses the body’s tendency to resist angular acceleration. For a disc, it is equal to half the product of mass of the disc and the square of radius of the disc. From the question, we are told that a small disc of diameter
Figure 1
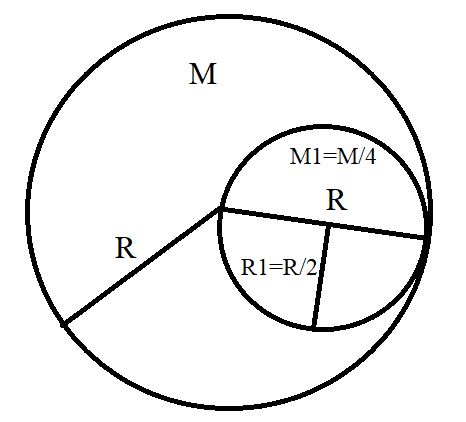
For the big disc, from which the small disc is cut out, let the moment of inertia be given by
where
Let this be equation 1.
Now, we are asked to find the moment of inertia about a perpendicular axis of the remaining part of the big disc after the small disc is cut out from it. Let us call this moment of inertia
Let this be equation 2.
Now, let us find the moment of inertia of the small disc
We have to use the parallel axis theorem here because the axis of the small disc is parallel to the axis of the big disc. Parallel axis theorem states that the moment of inertia of a body about an axis parallel to the body passing through its centre is equal to the sum of moment of inertia of body about the axis passing through the centre and product of mass of the body times the square of distance between the two axes i.e.
where
Let this be equation 3.
Let us calculate
where
Also, as provided in the question,
where
The moment of inertia at the centre of the small disc is given by
Now, let us substitute these values equation 3.
Let this be equation 4.
Moving on, let us substitute the values of equation 1 and equation 4 in equation 2. We have
Therefore, the moment of inertia of the remaining disc about a perpendicular axis passing through the centre, after the small disc is cut out from the big disc is equal to
Hence, the correct option is B.
Note:
In this question, we are required to find the moment of inertia of the remaining part of the disc about a perpendicular axis passing through the centre. From this, we can understand that the axis of the small disc, which is cut out, has an axis parallel to the big disc. In such a case, we have to use the parallel axis theorem. Students might have the tendency to use perpendicular axis theorem just because they see the word perpendicular axis in the question. Clearly understanding the question can easily sort out such problems.
Complete step by step answer:
Moment of inertia of a body is a quantity which expresses the body’s tendency to resist angular acceleration. For a disc, it is equal to half the product of mass of the disc and the square of radius of the disc. From the question, we are told that a small disc of diameter
Figure 1

For the big disc, from which the small disc is cut out, let the moment of inertia be given by
where
Let this be equation 1.
Now, we are asked to find the moment of inertia about a perpendicular axis of the remaining part of the big disc after the small disc is cut out from it. Let us call this moment of inertia
Let this be equation 2.
Now, let us find the moment of inertia of the small disc
We have to use the parallel axis theorem here because the axis of the small disc is parallel to the axis of the big disc. Parallel axis theorem states that the moment of inertia of a body about an axis parallel to the body passing through its centre is equal to the sum of moment of inertia of body about the axis passing through the centre and product of mass of the body times the square of distance between the two axes i.e.
where
Let this be equation 3.
Let us calculate
where
Also, as provided in the question,
where
The moment of inertia at the centre of the small disc is given by
Now, let us substitute these values equation 3.
Let this be equation 4.
Moving on, let us substitute the values of equation 1 and equation 4 in equation 2. We have
Therefore, the moment of inertia of the remaining disc about a perpendicular axis passing through the centre, after the small disc is cut out from the big disc is equal to
Hence, the correct option is B.
Note:
In this question, we are required to find the moment of inertia of the remaining part of the disc about a perpendicular axis passing through the centre. From this, we can understand that the axis of the small disc, which is cut out, has an axis parallel to the big disc. In such a case, we have to use the parallel axis theorem. Students might have the tendency to use perpendicular axis theorem just because they see the word perpendicular axis in the question. Clearly understanding the question can easily sort out such problems.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




