
Find the value of
Answer
399.3k+ views
Hint: In this question, we have to write the given trigonometric function in terms of fraction.
We know, that in a right- angled triangle, there are three sides, perpendicular, base and the hypotenuse.
And,
Complete answer:
Given trigonometric functions
To write these trigonometric functions in terms of fraction.
Consider a right- angled triangle,
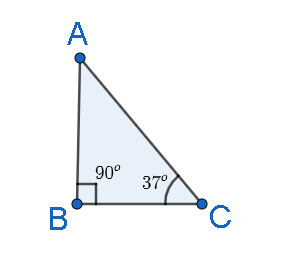
Then, we have, using angle sum property of a triangle, that,
Now, let length of side
Now, we know,
Similarly, for angle
Now, for angle
Similarly, for angle
Note:
It is not necessary to choose the lengths of sides of the triangle to be
If
For any angle, say
We know, that in a right- angled triangle, there are three sides, perpendicular, base and the hypotenuse.
And,
Complete answer:
Given trigonometric functions
To write these trigonometric functions in terms of fraction.
Consider a right- angled triangle,

Then, we have, using angle sum property of a triangle, that,
Now, let length of side
Now, we know,
Similarly, for angle
Now, for angle
Similarly, for angle
Note:
It is not necessary to choose the lengths of sides of the triangle to be
If
For any angle, say
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




