Answer
394.8k+ views
Hint: In order to solve this question, we have to apply the section formula which is if point $ P(x,y,z) $ divides the line segment joining the points $ A({{x}_{1}},{{y}_{1}},{{z}_{1}}) $ and $ B({{x}_{2}},{{y}_{2}},{{z}_{2}}) $ in the ratio $ m:n $ , then
$ \therefore x=\dfrac{m{{x}_{2}}+n{{x}_{1}}}{m+n} $ , $ y=\dfrac{m{{y}_{2}}+n{{y}_{1}}}{m+n} $ and $ z=\dfrac{m{{z}_{2}}+n{{z}_{1}}}{m+n} $
After applying the section formula, get the ratio in which $ P $ divides $ AB $ . With that ratio we have to find the harmonic conjugate of $ P $ and then get the final answer.
Complete step-by-step answer:
Let’s suppose that point $ P $ divides the line segment $ AB $ in the ratio $ k:1 $ .
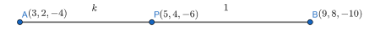
In order to find the value of $ k $ we have to apply a section formula.
According to section formula if point $ P(x,y,z) $ divides the line segment joining the points $ A({{x}_{1}},{{y}_{1}},{{z}_{1}}) $ and $ B({{x}_{2}},{{y}_{2}},{{z}_{2}}) $ in the ratio $ k:1 $ , then
$ \therefore x=\dfrac{k{{x}_{2}}+{{x}_{1}}}{k+1} $ , $ y=\dfrac{k{{y}_{2}}+{{y}_{1}}}{k+1} $ and $ z=\dfrac{k{{z}_{2}}+{{z}_{1}}}{k+1} $
Using the same logic, we have $ P(5,4,-6) $ , $ A(3,2,-4) $ and $ B(9,8,-10) $ , applying the section formula, we get
$ \Rightarrow 5=\dfrac{9k+3}{k+1} $
$ \Rightarrow 5k+5=9k+3 $
$ \Rightarrow 4k=2 $
$ \Rightarrow k=\dfrac{2}{4}=\dfrac{1}{2} $
Hence, $ P $ divides the line segment $ AB $ in the ratio $ \dfrac{1}{2}:1 $ , or we can say that $ 1:2 $ .
Now, let’s suppose $ Q(a,b,c) $ is the harmonic conjugate of $ P $ .
If $ P $ and $ Q $ are the harmonic conjugate, that means the ratio in which $ P $ divides the line segment $ AB $ internally $ Q $ also have to divide it in that ratio externally.
So, now $ Q $ divides the line segment $ AB $ in the ratio $ 1:2 $ externally.
Externally division means if point $ Q(x,y,z) $ divides the line segment joining the points $ A({{x}_{1}},{{y}_{1}},{{z}_{1}}) $ and $ B({{x}_{2}},{{y}_{2}},{{z}_{2}}) $ externally in the ratio $ m:n $ , then
$ \therefore x=\dfrac{m{{x}_{2}}-n{{x}_{1}}}{m-n} $ , $ y=\dfrac{m{{y}_{2}}-n{{y}_{1}}}{m-n} $ and $ z=\dfrac{m{{z}_{2}}-n{{z}_{1}}}{m-n} $
Using the same concept, we get
$ \Rightarrow a=\dfrac{9\times 1-2\times 3}{1-2}=\dfrac{9-6}{-1}=-3 $
$ \Rightarrow b=\dfrac{8\times 1-2\times 2}{1-2}=\dfrac{8-4}{-1}=-4 $
$ \Rightarrow c=\dfrac{-10\times 1-2\times -4}{1-2}=\dfrac{-10+8}{-1}=2 $
Hence, $ Q(-3,-4,2) $ .
So, the harmonic conjugate of $ P $ is $ (-3,-4,2) $ .
Note: In this question we just have to understand the difference between external and internal division of a line segment. First we have to apply internal division in order to find the ratio and then apply the external division formula to get the harmonic conjugate. Students often confuse external and internal division so apply the formulas carefully.
$ \therefore x=\dfrac{m{{x}_{2}}+n{{x}_{1}}}{m+n} $ , $ y=\dfrac{m{{y}_{2}}+n{{y}_{1}}}{m+n} $ and $ z=\dfrac{m{{z}_{2}}+n{{z}_{1}}}{m+n} $
After applying the section formula, get the ratio in which $ P $ divides $ AB $ . With that ratio we have to find the harmonic conjugate of $ P $ and then get the final answer.
Complete step-by-step answer:
Let’s suppose that point $ P $ divides the line segment $ AB $ in the ratio $ k:1 $ .
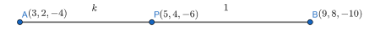
In order to find the value of $ k $ we have to apply a section formula.
According to section formula if point $ P(x,y,z) $ divides the line segment joining the points $ A({{x}_{1}},{{y}_{1}},{{z}_{1}}) $ and $ B({{x}_{2}},{{y}_{2}},{{z}_{2}}) $ in the ratio $ k:1 $ , then
$ \therefore x=\dfrac{k{{x}_{2}}+{{x}_{1}}}{k+1} $ , $ y=\dfrac{k{{y}_{2}}+{{y}_{1}}}{k+1} $ and $ z=\dfrac{k{{z}_{2}}+{{z}_{1}}}{k+1} $
Using the same logic, we have $ P(5,4,-6) $ , $ A(3,2,-4) $ and $ B(9,8,-10) $ , applying the section formula, we get
$ \Rightarrow 5=\dfrac{9k+3}{k+1} $
$ \Rightarrow 5k+5=9k+3 $
$ \Rightarrow 4k=2 $
$ \Rightarrow k=\dfrac{2}{4}=\dfrac{1}{2} $
Hence, $ P $ divides the line segment $ AB $ in the ratio $ \dfrac{1}{2}:1 $ , or we can say that $ 1:2 $ .
Now, let’s suppose $ Q(a,b,c) $ is the harmonic conjugate of $ P $ .
If $ P $ and $ Q $ are the harmonic conjugate, that means the ratio in which $ P $ divides the line segment $ AB $ internally $ Q $ also have to divide it in that ratio externally.
So, now $ Q $ divides the line segment $ AB $ in the ratio $ 1:2 $ externally.
Externally division means if point $ Q(x,y,z) $ divides the line segment joining the points $ A({{x}_{1}},{{y}_{1}},{{z}_{1}}) $ and $ B({{x}_{2}},{{y}_{2}},{{z}_{2}}) $ externally in the ratio $ m:n $ , then
$ \therefore x=\dfrac{m{{x}_{2}}-n{{x}_{1}}}{m-n} $ , $ y=\dfrac{m{{y}_{2}}-n{{y}_{1}}}{m-n} $ and $ z=\dfrac{m{{z}_{2}}-n{{z}_{1}}}{m-n} $
Using the same concept, we get
$ \Rightarrow a=\dfrac{9\times 1-2\times 3}{1-2}=\dfrac{9-6}{-1}=-3 $
$ \Rightarrow b=\dfrac{8\times 1-2\times 2}{1-2}=\dfrac{8-4}{-1}=-4 $
$ \Rightarrow c=\dfrac{-10\times 1-2\times -4}{1-2}=\dfrac{-10+8}{-1}=2 $
Hence, $ Q(-3,-4,2) $ .
So, the harmonic conjugate of $ P $ is $ (-3,-4,2) $ .
Note: In this question we just have to understand the difference between external and internal division of a line segment. First we have to apply internal division in order to find the ratio and then apply the external division formula to get the harmonic conjugate. Students often confuse external and internal division so apply the formulas carefully.
Recently Updated Pages
Assertion The resistivity of a semiconductor increases class 13 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

How do you arrange NH4 + BF3 H2O C2H2 in increasing class 11 chemistry CBSE

Is H mCT and q mCT the same thing If so which is more class 11 chemistry CBSE

What are the possible quantum number for the last outermost class 11 chemistry CBSE

Is C2 paramagnetic or diamagnetic class 11 chemistry CBSE

Trending doubts
State the differences between manure and fertilize class 8 biology CBSE

Why are xylem and phloem called complex tissues aBoth class 11 biology CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Difference Between Plant Cell and Animal Cell

What would happen if plasma membrane ruptures or breaks class 11 biology CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

What precautions do you take while observing the nucleus class 11 biology CBSE

What would happen to the life of a cell if there was class 11 biology CBSE

Change the following sentences into negative and interrogative class 10 english CBSE



