
Find the position of nodes and antinodes
Answer
506.1k+ views
2 likes
Hint: A node is a point along with a standing wave where the amplitude of the wave is minimum. For example, the two ends of a string vibrating is the node. At the point of the node, the particle of the standing wave does not vibrate. The opposite of the node is anti-node, so anti-node is a point where the amplitude of the standing wave is maximum. First, write down the wave equation of a stationary wave or standing wave. From that wave equation calculate the condition for which the amplitude is maximum and minimum which will give the conditions for nodes and anti-nodes. Compare that condition with the options to get the correct answer.
Formulas used: The equation of a wave is given by
Complete step-by-step solution:
First, we have to create a standing wave and see what will be the positions of nodes and anti-nodes.
Consider two sinusoidal waves of equal amplitude and frequency travelling along a long string in opposite directions. The wave travelling along positive X-direction can be represented as
The wave travelling along negative X-direction can be represented as
According to the principle of superposition, the resultant wave is given by
This equation represents a stationary wave. It cannot represent a progressive wave because the argument of any of its trigonometric functions does not contain the combination
Obviously in case of a stationary wave, the amplitude of oscillation is not same for all the particles. It varies harmonically with the location
The amplitude will be zero at points, where
These positions of zero amplitude are called nodes. Clearly, the separation between two consecutive nodes is
The amplitude will have a maximum value of
These positions of maximum amplitude are called antinodes. Clearly, the antinodes are separated by
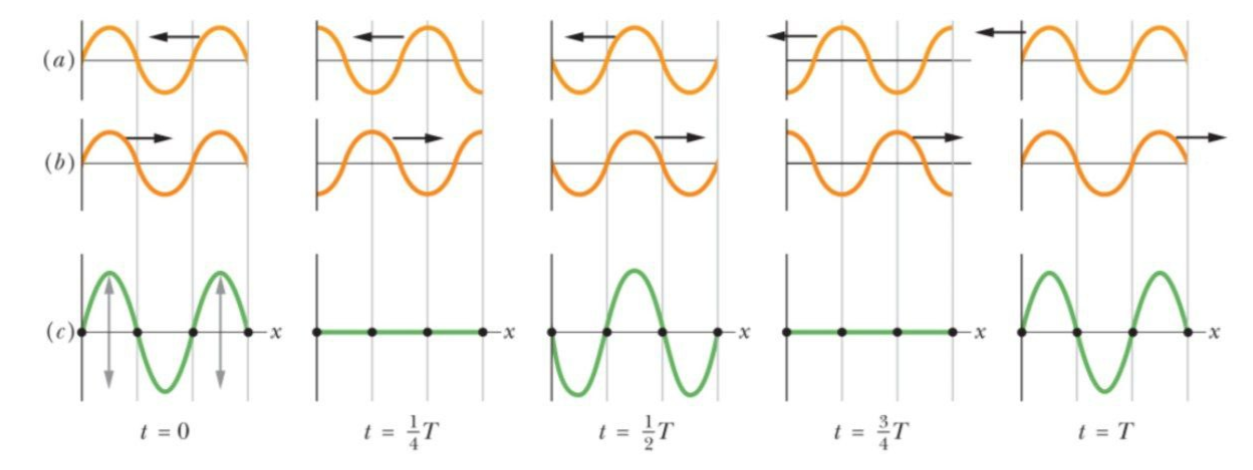
The figure represents five instants of the wave. The figure (a) and figure(b) represent the two identical wave. And figure (c) represents the resultant of the wave (a) and wave (b).
At
So the anti-nodes are situated at the exact midpoint of the nodes. From the options only option, only option C satisfies this condition. So the correct option is C.
Note: The changes of the wave with the time
At the instants
Thus at these instants the displacement
At the instants
Thus at these instants the displacement
Formulas used: The equation of a wave is given by
Complete step-by-step solution:
First, we have to create a standing wave and see what will be the positions of nodes and anti-nodes.
Consider two sinusoidal waves of equal amplitude and frequency travelling along a long string in opposite directions. The wave travelling along positive X-direction can be represented as
The wave travelling along negative X-direction can be represented as
According to the principle of superposition, the resultant wave is given by
This equation represents a stationary wave. It cannot represent a progressive wave because the argument of any of its trigonometric functions does not contain the combination
Obviously in case of a stationary wave, the amplitude of oscillation is not same for all the particles. It varies harmonically with the location
The amplitude will be zero at points, where
These positions of zero amplitude are called nodes. Clearly, the separation between two consecutive nodes is
The amplitude will have a maximum value of
These positions of maximum amplitude are called antinodes. Clearly, the antinodes are separated by

The figure represents five instants of the wave. The figure (a) and figure(b) represent the two identical wave. And figure (c) represents the resultant of the wave (a) and wave (b).
At
So the anti-nodes are situated at the exact midpoint of the nodes. From the options only option, only option C satisfies this condition. So the correct option is C.
Note: The changes of the wave with the time
At the instants
Thus at these instants the displacement
At the instants
Thus at these instants the displacement
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

How do I convert ms to kmh Give an example class 11 physics CBSE

Where can free central placentation be seen class 11 biology CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE




