
How do we find the latus rectum of the parabola
Answer
414.3k+ views
Hint: We are given the equation of a parabola and we have to find the length of its latus rectum.
So, first, we will find the end coordinates of the latus rectum, and then using the distance formula, we will find its length.
General equation of parabola:
Distance between the points
Complete step by step answer:
Given the equation of the parabola:
To find the length of the latus rectum of the parabola.
Let the endpoints of the latus rectum of the parabola be
First, we will find these coordinates then their length.
Let
Taking square root, gives,
Thus, the endpoints of the latus rectum of the parabola are
Now, the length of the latus rectum is given
Putting values, we get,
On solving, we get, length of the latus rectum
Now, comparing the given equation with the general equation of a parabola, we get,
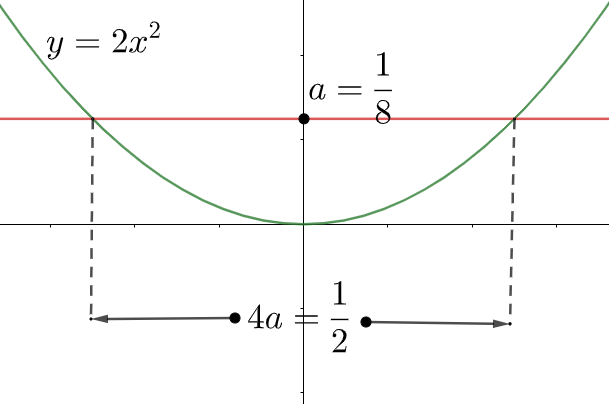
Therefore, the length of the latus rectum of the parabola
Note:In general, the length of the latus rectum of a parabola is
In the conic section, the latus rectum is the chord drawn from the focus and is parallel to the directrix.
In general, there are 4 types of parabolas, with equations
So, first, we will find the end coordinates of the latus rectum, and then using the distance formula, we will find its length.
General equation of parabola:
Distance between the points
Complete step by step answer:
Given the equation of the parabola:
To find the length of the latus rectum of the parabola.
Let the endpoints of the latus rectum of the parabola be
First, we will find these coordinates then their length.
Let
Taking square root, gives,
Thus, the endpoints of the latus rectum of the parabola are
Now, the length of the latus rectum is given
Putting values, we get,
On solving, we get, length of the latus rectum
Now, comparing the given equation with the general equation of a parabola, we get,

Therefore, the length of the latus rectum of the parabola
Note:In general, the length of the latus rectum of a parabola is
In the conic section, the latus rectum is the chord drawn from the focus and is parallel to the directrix.
In general, there are 4 types of parabolas, with equations
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

1 litre is equivalent to A 1000mL B 100cm3 C 10mL D class 11 physics CBSE

A car travels 100 km at a speed of 60 kmh and returns class 11 physics CBSE

Name the Largest and the Smallest Cell in the Human Body ?

Explain zero factorial class 11 maths CBSE

In tea plantations and hedge making gardeners trim class 11 biology CBSE




