
Find the height of the cone if the slant height h is 34cm and base diameter is 32cm.
Answer
513k+ views
1 likes
Hint: First, we will consider the height of the cone as h1. Then we will find the radius of the cone by using the formula
Complete step-by-step answer:
Here, we will first draw the diagram of cones with all dimensions given. So, diagram is as given below:
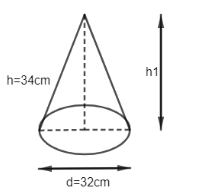
We have slant height h, diameter d as 32cm and we have assumed height of cone to be h1.
So, to find height of cone, the formula to be used is given as
On putting the value, we get as
Radius
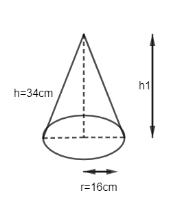
Now, again substituting the value in the equation
Now, we will take squares on both sides, we will get as
Now, we will make h1 as subject so, we get as
Now, this above equation is in form
On further solving, we get as
Now, taking square root on both sides, we get as
Now, we can write this as
Thus, the height of the cone is 30cm.
Note: Students should remember one formula which is used to find either radius, height or slant height which is given as
Complete step-by-step answer:
Here, we will first draw the diagram of cones with all dimensions given. So, diagram is as given below:

We have slant height h, diameter d as 32cm and we have assumed height of cone to be h1.
So, to find height of cone, the formula to be used is given as
On putting the value, we get as
Radius

Now, again substituting the value in the equation
Now, we will take squares on both sides, we will get as
Now, we will make h1 as subject so, we get as
Now, this above equation is in form
On further solving, we get as
Now, taking square root on both sides, we get as
Now, we can write this as
Thus, the height of the cone is 30cm.
Note: Students should remember one formula which is used to find either radius, height or slant height which is given as
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Find the largest number which divides 615 and 963 leaving class 9 maths CBSE

In a morning walk three persons step off together -class-9-maths-CBSE

When did South Africa become independent A 16 April class 9 social science CBSE

The president of the constituent assembly was A Dr class 9 social science CBSE

The French Revolution popularised many symbols Each class 9 social science CBSE

Why did Gujarat witness a sunrise 2 hours after Arunachal Pradesh?




