
Find the factors of 330.
A.
B.
C.
D.
Answer
489k+ views
Hint: Here, we will find the factors of the given number using the concept of prime factorization. Prime factorization is a method in which a number is factorized to get factors as prime numbers. Factors are defined as the whole number multiplied by a number to get another number.
Complete step-by-step answer:
We are given a number 330.
We will find the factors of 330 by using the method of prime factorization.
We will follow the following steps has to perform doing prime factorization:
First, we will divide the number by the smallest prime number which divides the number exactly.
We will divide the quotient again by the smallest or the next smallest prime number if it is not exactly divisible by the smallest prime number.
We divide the process again and again till the quotient becomes 1.
We multiply the factors.
So, using the steps, we get
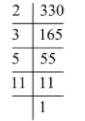
Thus, the factors of 330 are 2, 3, 5, and 11. As the factors are prime numbers, therefore we cannot factorize it further.
Therefore, the factors of 330 are
Note: We should remember that we should use only the prime factors. the product should be the number itself. We can also use the factor tree method.
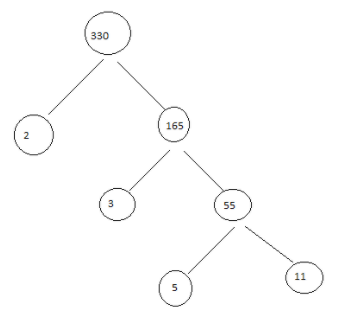
In factor tree method, the given number has to be multiplied by a prime factor and a composite factor. The composite factor has to be factorized by a prime factor and a composite factor. This has to be continued till all the factors become the prime factors. Each number is a factor of itself and The number 1 is a factor of every number. Thus the number 1 can be neglected. The product should be the number itself. Thus the numbers become the factors.
Complete step-by-step answer:
We are given a number 330.
We will find the factors of 330 by using the method of prime factorization.
We will follow the following steps has to perform doing prime factorization:
First, we will divide the number by the smallest prime number which divides the number exactly.
We will divide the quotient again by the smallest or the next smallest prime number if it is not exactly divisible by the smallest prime number.
We divide the process again and again till the quotient becomes 1.
We multiply the factors.
So, using the steps, we get

Thus, the factors of 330 are 2, 3, 5, and 11. As the factors are prime numbers, therefore we cannot factorize it further.
Therefore, the factors of 330 are
Note: We should remember that we should use only the prime factors. the product should be the number itself. We can also use the factor tree method.

In factor tree method, the given number has to be multiplied by a prime factor and a composite factor. The composite factor has to be factorized by a prime factor and a composite factor. This has to be continued till all the factors become the prime factors. Each number is a factor of itself and The number 1 is a factor of every number. Thus the number 1 can be neglected. The product should be the number itself. Thus the numbers become the factors.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Convert 200 Million dollars in rupees class 7 maths CBSE

What is meant by Indian Standard Time Why do we need class 7 social science CBSE

Aeroplanes fly in which of the following layers of class 7 social science CBSE

What Is Indian Standard Time and what is its impor class 7 physics CBSE

Differentiate between map and globe class 7 social science CBSE

Collective noun a of sailors class 7 english CBSE





