
How do you find the exact value of
Answer
478.5k+ views
Hint:
Complete Step by Step Solution:
Consider the following right-angled triangle
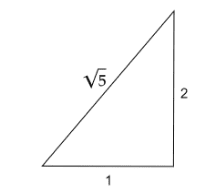
These sides of the triangle for
Opposite = 2, Adjacent = 1, and Hypotenuse =
However,
Therefore, we need a different method to represent this. Hence, we represent
This, however, can only be converged for
In order for this series to converge, we need to use:
Taking arctan on both sides of the equation, we get
Substituting the values of x in the above equation,
Simplifying the equation, we arrive at the conclusion,
Note:
Every trigonometric function has an inverse function. The inverse function works in a reverse manner. An inverse function is written with an arc in front of the name of the function, i.e., arcsine, arccos, arctan, etc.
Trigonometric functions can be applied to any angle including large and obtuse angles. However, inverse functions pose a problem because we will find many angles having the same tangent. For example,
Complete Step by Step Solution:
Consider the following right-angled triangle

These sides of the triangle for
Opposite = 2, Adjacent = 1, and Hypotenuse =
However,
Therefore, we need a different method to represent this. Hence, we represent
This, however, can only be converged for
In order for this series to converge, we need to use:
Taking arctan on both sides of the equation, we get
Substituting the values of x in the above equation,
Simplifying the equation, we arrive at the conclusion,
Note:
Every trigonometric function has an inverse function. The inverse function works in a reverse manner. An inverse function is written with an arc in front of the name of the function, i.e., arcsine, arccos, arctan, etc.
Trigonometric functions can be applied to any angle including large and obtuse angles. However, inverse functions pose a problem because we will find many angles having the same tangent. For example,
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




