
Find the electric potential at the axis of a uniformly charged disc and use the potential to find the electric field at the same point.
Answer
495.9k+ views
4 likes
Hint: First consider a small part of that disc that has a radius
Complete answer:
Electric field – It is the space around a charge where the electrostatic force of attraction or repulsion can be felt by other charges and charged bodies.
Electric potential –Electric potential at any point is the work done in bringing a unit positive charge in an electric point to that point.
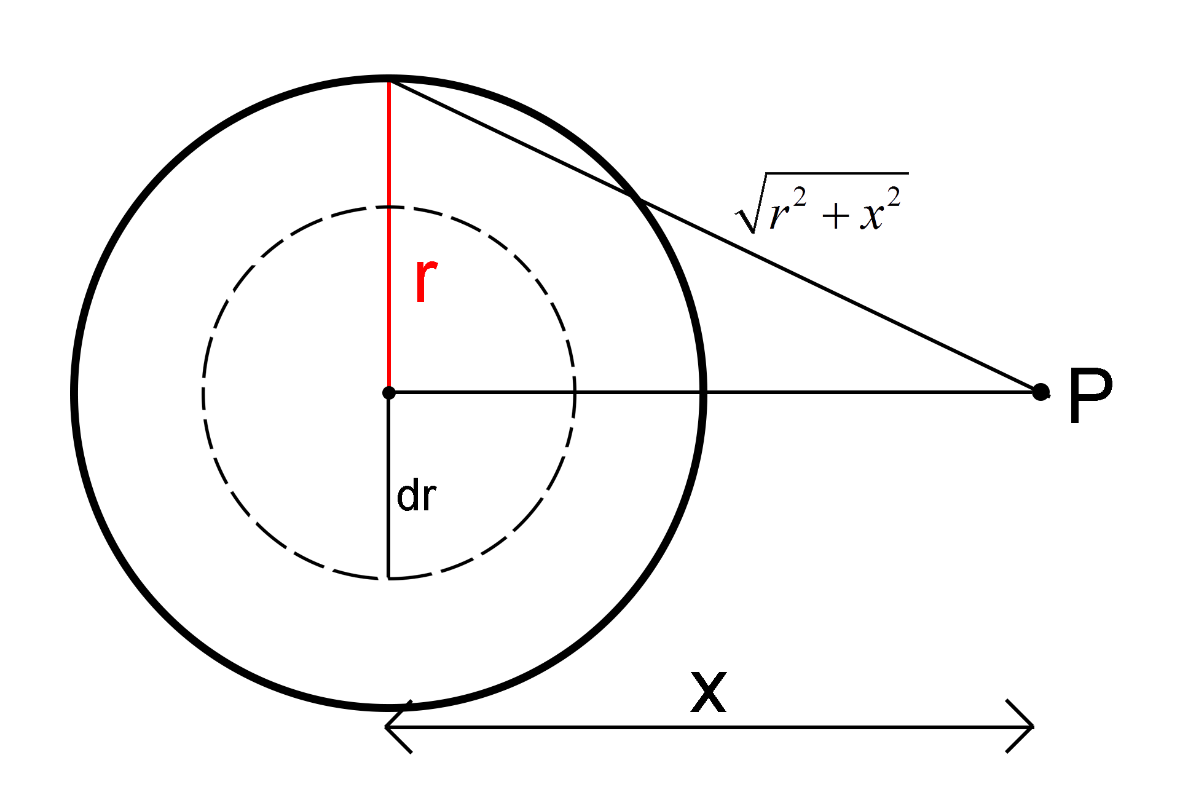
Let’s consider a charged disc of a radius
To calculate the electric potential at point P due to the charged disc, consider a small part of the disc which is of a radius
So, the charge
We know the electric potential at a point is given by
Here,
So, the electric point at point P due to the charged disc of a radius
Integrating the equation, we get
We know that the magnitude of the electric field at any point is given by the following equation
So, the magnitude of the electric field at point P due to a uniformly charged disc is
Note:
By the equation for electric potential and the magnitude of the electric field, i.e.
Complete answer:
Electric field – It is the space around a charge where the electrostatic force of attraction or repulsion can be felt by other charges and charged bodies.
Electric potential –Electric potential at any point is the work done in bringing a unit positive charge in an electric point to that point.

Let’s consider a charged disc of a radius
To calculate the electric potential at point P due to the charged disc, consider a small part of the disc which is of a radius
So, the charge
We know the electric potential at a point is given by
Here,
So, the electric point at point P due to the charged disc of a radius
Integrating the equation, we get
We know that the magnitude of the electric field at any point is given by the following equation
So, the magnitude of the electric field at point P due to a uniformly charged disc is
Note:
By the equation for electric potential and the magnitude of the electric field, i.e.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

What are saprophytes Give two examples class 12 biology CBSE

How do you convert from joules to electron volts class 12 physics CBSE

Mirage is a phenomena due to A Refraction of light class 12 physics CBSE

Why did French artist Frederic sorrieu prepare a series class 12 social science CBSE

What is Nutrition Explain Diff Type of Nutrition ?




