
Find the area of the minor segment of a circle of radius 42 cm, if the length of the corresponding arc is 44 cm.
Answer
504k+ views
Hint: In this sum, we will first draw figure for better understanding. After that, we will find angles subtended by an arc of the given length. For finding the area of the segment, we need to find areas of sector and area of a triangle formed. We can find the area of the sector by using angles found. For finding the area of the triangle we will use basic formula but for finding base and height we will use congruency, right-angled triangle properties.
Complete step-by-step solution
Formulas which we will use are:
I)
II) Area of segment = Area of the sector - Area of a triangle.
III)
IV)
Let us draw the figure first:
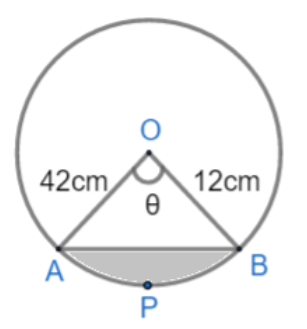
Now, let us find angle subtended by arc with help of length of arc 44 cm, radius 42 cm. As we know,
Putting the values we get:
As we can see from diagram, area of segment APB = Area of sector OAPB - Area of triangle OAB . . . . . . . . . (2) so, let us first find area of sector OAPB and area of triangle OAB. Given radius r = 42 cm.
Putting value of
But we don't know values of base or height. So let us find that,
Draw
Therefore,
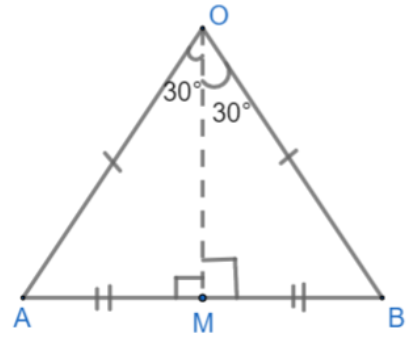
In
OA = OB as both is the radius of the same circle.
OM = OM which is common in both triangles.
Therefore, by RHS congruency criterion,
By using CPCT (corresponding parts of congruent triangle) we can say,
Therefore,
As we have find
Therefore,
Also,
Now from
Also,
From (3) AB = 2AM
Therefore, putting value of AM, we get:
AB = 42 cm
Also,
So, we have found length of base and perpendicular for triangle AOB.
From (2) as we know, area of segment APB = area of sector OAPB - area of triangle OAB.
We have,
Note: Students should not get confused with length of arc or length of chord such that, by two points. Students should always draw diagrams for better understanding. Also, don't get confused with formulas of
Complete step-by-step solution
Formulas which we will use are:
I)
II) Area of segment = Area of the sector - Area of a triangle.
III)
IV)
Let us draw the figure first:

Now, let us find angle subtended by arc with help of length of arc 44 cm, radius 42 cm. As we know,
Putting the values we get:
As we can see from diagram, area of segment APB = Area of sector OAPB - Area of triangle OAB . . . . . . . . . (2) so, let us first find area of sector OAPB and area of triangle OAB. Given radius r = 42 cm.
Putting value of
But we don't know values of base or height. So let us find that,
Draw
Therefore,

In
OA = OB as both is the radius of the same circle.
OM = OM which is common in both triangles.
Therefore, by RHS congruency criterion,
By using CPCT (corresponding parts of congruent triangle) we can say,
Therefore,
As we have find
Therefore,
Also,
Now from
Also,
From (3) AB = 2AM
Therefore, putting value of AM, we get:
AB = 42 cm
Also,
So, we have found length of base and perpendicular for triangle AOB.
From (2) as we know, area of segment APB = area of sector OAPB - area of triangle OAB.
We have,
Note: Students should not get confused with length of arc or length of chord such that, by two points. Students should always draw diagrams for better understanding. Also, don't get confused with formulas of
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹ per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




