
Find the angle of intersection of two circles?

Answer
499.5k+ views
3 likes
Hint: To solve this question, we will, first of all, compute the centre and radius of both the circles using the formula
Complete step-by-step solution:
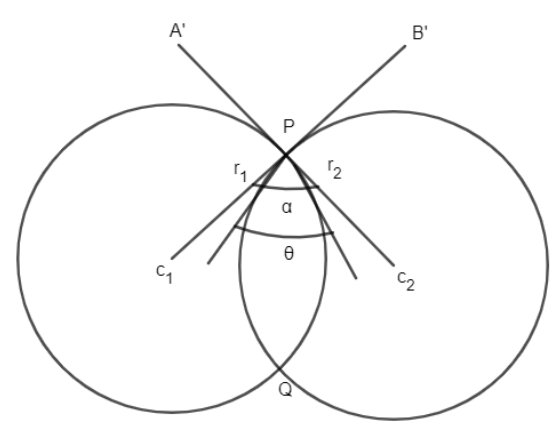
We are given the two circles below.
They are given as

If the circles intersect at P, then the angle
where a, b, h, g, f and c are constants and the radius of the circle is given by
And the centre of the circle is given by
Comparing this theory with our given equation (i) and equation (ii) of the circle, we have the centre as
And radius is given as,
Clearly, as visible by the above diagram, the distance ‘d’ between the circles is given by
The formula of the distance between the two points
Using this formula to find the distance
Now considering the triangle
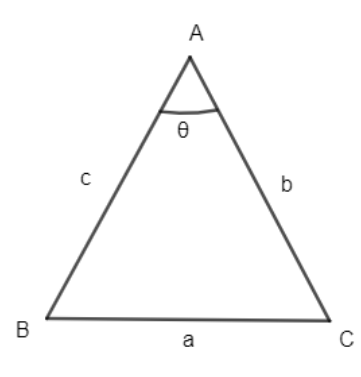
Now, if triangle ABC is given as below, where the angle
Applying this formula on the angle
where
Now,
Now, because
Finally, we have a full circle angle that is
Now, as
Hence, the angle between the circle is given by
Note: Because
Complete step-by-step solution:
We are given the two circles below.
They are given as

If the circles intersect at P, then the angle
where a, b, h, g, f and c are constants and the radius of the circle is given by
And the centre of the circle is given by
Comparing this theory with our given equation (i) and equation (ii) of the circle, we have the centre as
And radius is given as,
Clearly, as visible by the above diagram, the distance ‘d’ between the circles is given by
The formula of the distance between the two points
Using this formula to find the distance
Now considering the triangle

Now, if triangle ABC is given as below, where the angle
Applying this formula on the angle
where
Now,
Now, because
Finally, we have a full circle angle that is
Now, as
Hence, the angle between the circle is given by
Note: Because
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Write examples of herbivores carnivores and omnivo class 10 biology CBSE

Difference between mass and weight class 10 physics CBSE

List out three methods of soil conservation

Leap year has days A 365 B 366 C 367 D 368 class 10 maths CBSE

How does Tommy describe the old kind of school class 10 english CBSE




