
How do you find the amplitude, period, vertical and phase shift and graph for
Answer
472.2k+ views
Hint: Convert the given angle in degrees into radian and write the function as
Complete step by step solution:
Here, we have been provided with the function
Now, the general form of the cosine function is given as
1. If
2. If ‘a’ is negative then the function is reflected about the x – axis.
3. If
4. If ‘b’ is negative then the function is reflected about y – axis.
5. If ‘c’ is negative then the function is shifted
6. If ‘d’ is negative then the function is shifted
Now, on comparing the given function
(i) Amplitude: - Amplitude of a function is defined as the minimum and maximum value that a function can approach. Clearly, the value of ‘a’ is 1 unit. Therefore the amplitude of the given function is 1 unit. Therefore, there will not be any vertical stretch or compression in the function provided.
(ii) Time period: - Time period of a function is defined as the interval of x after which the value of the function starts repeating itself. It is also simply called the period and the function is known as periodic function. The period of the function
Now, we know that the period of
Therefore, the period of the provided function is
(iii) Phase shift: - Phase shift of a function is defined as how far a function is shifted horizontally from the usual position. Clearly, the value of c is
(iv) Vertical shift: - Vertical shift of a function is defined as how far a function is shifted vertically from the usual position. Clearly, the value of d is 0, so the vertical shift is 0 units. Therefore, the function will not be shifted vertically.
Now, let us draw the graph of the function.
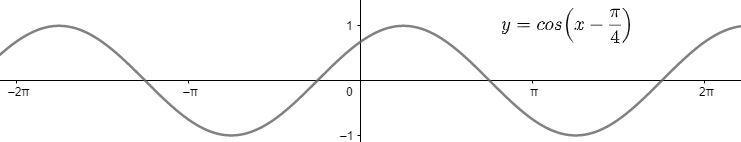
Note: You must remember the descriptions of the transform of the function otherwise it will be difficult to draw the graph. Note that the phase shift has no effect on time period. Time period changes only when the coefficient of x is changed in the function. The graphs of these functions are helpful in chapters such as integration where we have to find the area under the curve that is a part of a graph.
Complete step by step solution:
Here, we have been provided with the function
Now, the general form of the cosine function is given as
1. If
2. If ‘a’ is negative then the function is reflected about the x – axis.
3. If
4. If ‘b’ is negative then the function is reflected about y – axis.
5. If ‘c’ is negative then the function is shifted
6. If ‘d’ is negative then the function is shifted
Now, on comparing the given function
(i) Amplitude: - Amplitude of a function is defined as the minimum and maximum value that a function can approach. Clearly, the value of ‘a’ is 1 unit. Therefore the amplitude of the given function is 1 unit. Therefore, there will not be any vertical stretch or compression in the function provided.
(ii) Time period: - Time period of a function is defined as the interval of x after which the value of the function starts repeating itself. It is also simply called the period and the function is known as periodic function. The period of the function
Now, we know that the period of
Therefore, the period of the provided function is
(iii) Phase shift: - Phase shift of a function is defined as how far a function is shifted horizontally from the usual position. Clearly, the value of c is
(iv) Vertical shift: - Vertical shift of a function is defined as how far a function is shifted vertically from the usual position. Clearly, the value of d is 0, so the vertical shift is 0 units. Therefore, the function will not be shifted vertically.
Now, let us draw the graph of the function.

Note: You must remember the descriptions of the transform of the function otherwise it will be difficult to draw the graph. Note that the phase shift has no effect on time period. Time period changes only when the coefficient of x is changed in the function. The graphs of these functions are helpful in chapters such as integration where we have to find the area under the curve that is a part of a graph.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

A car travels 100 km at a speed of 60 kmh and returns class 11 physics CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE

In tea plantations and hedge making gardeners trim class 11 biology CBSE

A difference between diffusion and osmosis is a A semipermeable class 11 chemistry CBSE




