
Find number of solutions of the equation
Answer
516.9k+ views
Hint: To solve the question given above, we will first find out the range of the function
Complete step-by-step answer:
To start with, we will first find out the range of the function
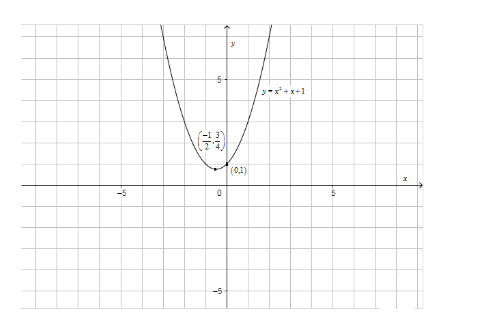
Now, we have to determine the value of
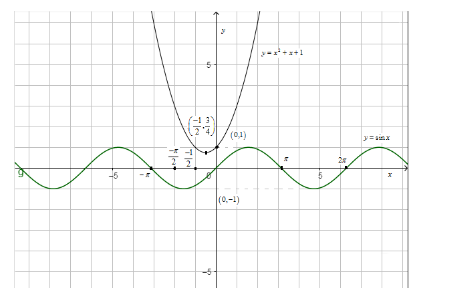
Thus, we can see that both the graphs do not intersect at all. Thus, there will be no solution of
Hence, there are 0 solutions of the equation
Note: he minima of
We know that if
On differentiating both sides, we have:
Now,
At
Complete step-by-step answer:
To start with, we will first find out the range of the function

Now, we have to determine the value of

Thus, we can see that both the graphs do not intersect at all. Thus, there will be no solution of
Hence, there are 0 solutions of the equation
Note: he minima of
We know that if
On differentiating both sides, we have:
Now,
At
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is insulin not administered orally to a diabetic class 12 biology CBSE

How do you convert from joules to electron volts class 12 physics CBSE

Why should a magnesium ribbon be cleaned before burning class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE

The current flowing through the resistor in a series class 12 physics CBSE




