
Figure shows grouping of identical cells each of emf
(A).
(B).
(C).
(D).


Answer
455.4k+ views
1 likes
Hint: Figure shows batteries connected in series and parallel combination. The combination of batteries is similar to the combination of resistances. Therefore, using formula for equivalent emf in series and equivalent resistances in series we can get a combination of batteries purely in parallel combination, we can solve the combination to calculate the equivalent emf and internal resistance in the circuit.
Formulas used:
Complete answer:
For all elements connected in series, the current passing through them is the same while for all elements connected in parallel the potential drop is the same. Batteries can also be connected in series or parallel combination.
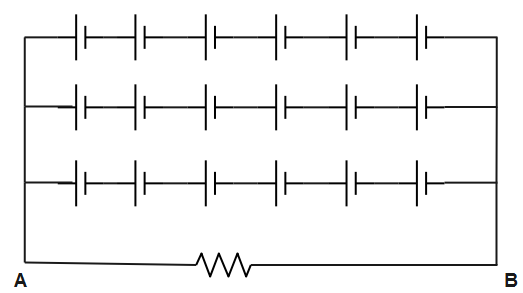
In the above figure, given that each battery has emf
For series combination of batteries, the equivalent emf is given by-
Similarly the equivalent internal resistance of batteries connected in series will be-
The equivalent emf of batteries connected in series in the figure will be-
The equivalent resistance of batteries connected in series will be-
Therefore, the equivalent resistance of batteries connected in series is
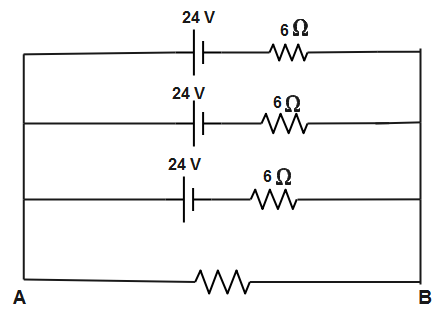
For all batteries connected in parallel, as the voltage is same in parallel, the equivalent emf will be
For internal resistances connected in parallel, the formula for equivalent internal resistances is-
Therefore, the equivalent internal resistance will be-
Therefore, the equivalent internal resistance will be
Therefore, the combination of batteries can be replaced by a battery of emf
Hence, the correct option is (D).
Note:
The emf is the potential developed by a battery. It is always greater than the potential difference. Potential difference is the difference of electric potential between two points in a circuit. Charge always flows from a point of high potential to a point of low potential. Flow of charge is called current.
Formulas used:
Complete answer:
For all elements connected in series, the current passing through them is the same while for all elements connected in parallel the potential drop is the same. Batteries can also be connected in series or parallel combination.

In the above figure, given that each battery has emf
For series combination of batteries, the equivalent emf is given by-
Similarly the equivalent internal resistance of batteries connected in series will be-
The equivalent emf of batteries connected in series in the figure will be-
The equivalent resistance of batteries connected in series will be-
Therefore, the equivalent resistance of batteries connected in series is

For all batteries connected in parallel, as the voltage is same in parallel, the equivalent emf will be
For internal resistances connected in parallel, the formula for equivalent internal resistances is-
Therefore, the equivalent internal resistance will be-
Therefore, the equivalent internal resistance will be
Therefore, the combination of batteries can be replaced by a battery of emf
Hence, the correct option is (D).
Note:
The emf is the potential developed by a battery. It is always greater than the potential difference. Potential difference is the difference of electric potential between two points in a circuit. Charge always flows from a point of high potential to a point of low potential. Flow of charge is called current.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

How do I convert ms to kmh Give an example class 11 physics CBSE

Where can free central placentation be seen class 11 biology CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE




