
Answer
386.1k+ views
Hint: First understand that the given numbers have to be divided by prime numbers which are also the factors of the numbers. Then convert the numbers into the product of its prime factors and write it as it is.
“Complete step-by-step answer:”
We are given some numbers and asked to express them as a product of their prime factors.
First we will understand what a prime factor is.
A prime factor is a prime number. In other words any of the prime numbers that will be multiplied to give the original number is a prime factor.
For example: $15=3\times 5$, here 3 and 5 are prime numbers and factors of 15.
We will find the prime factors by dividing the number by the prime number until its quotient is a prime number or 1.
In this question the numbers given are,
(i) 140 (ii) 156 (iii) 3825 (iv) 5005 (v) 7429
Let’s take 140,

Now 140 can be expressed as $2\times 2\times 5\times 7$.
So, $140={{2}^{2}}\times 5\times 7$
Let’s take 156,

Now 156 can be expressed as $2\times 2\times 3\times 13$.
So, $156={{2}^{2}}\times 3\times 13$.
Let’s take 3825.
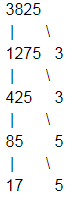
Now 3825 can be expressed as $3\times 3\times 5\times 5\times 17.$
So, $3825={{3}^{2}}\times {{5}^{2}}\times 17.$
Let’s take 5005,

Now 5005 can be expressed as $5\times 7\times 11\times 13.$
So, $5005=5\times 7\times 11\times 13.$
Let’s take 7429,
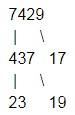
Now 7429 can be expressed as $17\times 19\times 23.$
So, $7429=17\times 19\times 23.$
So the required answer is
$\begin{align}
& 140={{2}^{2}}\times 5\times 7 \\
& 156={{2}^{2}}\times 3\times 13 \\
& 3825={{3}^{2}}\times {{5}^{2}}\times 17 \\
& 5005=5\times 7\times 11\times 13 \\
& 7429=17\times 19\times 23 \\
\end{align}$
Note: While dividing the students must be careful about calculations because any mistake will make your whole answer wrong. Students should choose their divisors which are prime numbers and should use their prime factors for division according to ascending order to avoid any kind of errors or mistakes in their answers.
“Complete step-by-step answer:”
We are given some numbers and asked to express them as a product of their prime factors.
First we will understand what a prime factor is.
A prime factor is a prime number. In other words any of the prime numbers that will be multiplied to give the original number is a prime factor.
For example: $15=3\times 5$, here 3 and 5 are prime numbers and factors of 15.
We will find the prime factors by dividing the number by the prime number until its quotient is a prime number or 1.
In this question the numbers given are,
(i) 140 (ii) 156 (iii) 3825 (iv) 5005 (v) 7429
Let’s take 140,

Now 140 can be expressed as $2\times 2\times 5\times 7$.
So, $140={{2}^{2}}\times 5\times 7$
Let’s take 156,

Now 156 can be expressed as $2\times 2\times 3\times 13$.
So, $156={{2}^{2}}\times 3\times 13$.
Let’s take 3825.
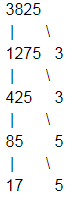
Now 3825 can be expressed as $3\times 3\times 5\times 5\times 17.$
So, $3825={{3}^{2}}\times {{5}^{2}}\times 17.$
Let’s take 5005,

Now 5005 can be expressed as $5\times 7\times 11\times 13.$
So, $5005=5\times 7\times 11\times 13.$
Let’s take 7429,
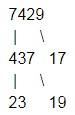
Now 7429 can be expressed as $17\times 19\times 23.$
So, $7429=17\times 19\times 23.$
So the required answer is
$\begin{align}
& 140={{2}^{2}}\times 5\times 7 \\
& 156={{2}^{2}}\times 3\times 13 \\
& 3825={{3}^{2}}\times {{5}^{2}}\times 17 \\
& 5005=5\times 7\times 11\times 13 \\
& 7429=17\times 19\times 23 \\
\end{align}$
Note: While dividing the students must be careful about calculations because any mistake will make your whole answer wrong. Students should choose their divisors which are prime numbers and should use their prime factors for division according to ascending order to avoid any kind of errors or mistakes in their answers.
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Mark and label the given geoinformation on the outline class 11 social science CBSE

When people say No pun intended what does that mea class 8 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

Give an account of the Northern Plains of India class 9 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

Give 10 examples for herbs , shrubs , climbers , creepers

Difference Between Plant Cell and Animal Cell

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Change the following sentences into negative and interrogative class 10 english CBSE

How do you graph the function fx 4x class 9 maths CBSE

Write a letter to the principal requesting him to grant class 10 english CBSE



