
How does the period of an oscillation in a spring-mass system depend on mass and amplitude? How can I express this graphically for period as a function of mass, period as a function of the square root of the mass, and period as a function of amplitude?
Answer
446.4k+ views
Hint: In order to answer the following question, we will be describing the most basic spring-mass system. We will derive the equation of the time period of oscillations of such a system. Finally, we will represent the graph of time period with different types of variables as asked in the question.
Formula used:
Time period of oscillation:
Where
Complete step by step answer:
First of all, we will draw a simple diagram of a spring-mass system.
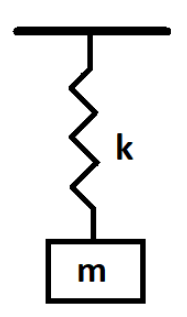
As we can see from the figure, a mass
If we square both sides of the above equation, the equation becomes
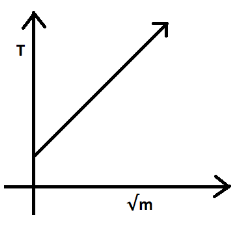
The above equation implies that the time period of oscillation is directly proportional to the square root of mass. It also states that the time period is independent of the amplitude.Therefore, if we plot a graph of

Now, if we plot a graph of
Lastly, the graph of
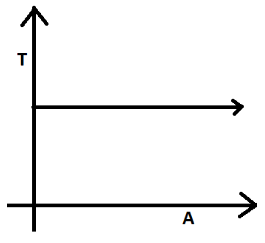
Note:It is very important to keep in mind that the graph of
Formula used:
Time period of oscillation:
Where
Complete step by step answer:
First of all, we will draw a simple diagram of a spring-mass system.

As we can see from the figure, a mass
If we square both sides of the above equation, the equation becomes
The above equation implies that the time period of oscillation is directly proportional to the square root of mass. It also states that the time period is independent of the amplitude.Therefore, if we plot a graph of

Now, if we plot a graph of

Lastly, the graph of

Note:It is very important to keep in mind that the graph of
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




