
How does soh cah toa apply to a right angle triangle ?
Answer
469.5k+ views
Hint: In order to find a solution to this problem, we have to be able to recognize what type of question it is. As we can see, this is a problem of trigonometric function. It states the formula that is used in trigonometric function and that is applied to a right angle triangle.
Complete step by step solution:
In geometry, trigonometric functions are the functions that are used to find the unknown angle or side of a right-angled triangle.
There are three basic trigonometric functions: Sine, Cosine and Tangent.
The angles of sine, cosine, and tangent are the primary classification of functions of trigonometry. And the three functions which are cotangent, secant and cosecant can be derived from the primary functions. So Basically, the other three functions are often used as compared to the primary trigonometric functions.
If
Now consider the right angle triangle ABC. Let angle A be angle
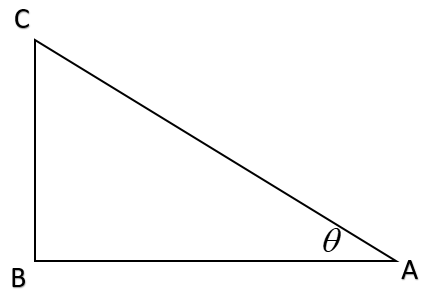
That is can be denoted as:
We can remember this trigonometric functions by making use of mnemonics that is,
soa, cah, toa
This means,
soh:
Sin = Opposite over Hypotenuse
cah:
Cos = Adjacent over Hypotenuse
toa:
Tan = Opposite over Adjacent
Therefore, these are a set of mnemonics to help remember how to derive the basic trigonometric functions for a (non-right) angle of a right triangle.
Note:
Trigonometric functions are also known as a Circular Functions can be simply defined as the functions of an angle of a triangle. It means that the relationship between the angles and sides of a triangle are given by these trig functions. The basic trigonometric functions are sine, cosine, tangent, cotangent, secant and cosecant.
Also, the above mnemonics can only apply to right angle triangles.
Complete step by step solution:
In geometry, trigonometric functions are the functions that are used to find the unknown angle or side of a right-angled triangle.
There are three basic trigonometric functions: Sine, Cosine and Tangent.
The angles of sine, cosine, and tangent are the primary classification of functions of trigonometry. And the three functions which are cotangent, secant and cosecant can be derived from the primary functions. So Basically, the other three functions are often used as compared to the primary trigonometric functions.
If
Now consider the right angle triangle ABC. Let angle A be angle

That is can be denoted as:
We can remember this trigonometric functions by making use of mnemonics that is,
soa, cah, toa
This means,
soh:
Sin = Opposite over Hypotenuse
cah:
Cos = Adjacent over Hypotenuse
toa:
Tan = Opposite over Adjacent
Therefore, these are a set of mnemonics to help remember how to derive the basic trigonometric functions for a (non-right) angle of a right triangle.
Note:
Trigonometric functions are also known as a Circular Functions can be simply defined as the functions of an angle of a triangle. It means that the relationship between the angles and sides of a triangle are given by these trig functions. The basic trigonometric functions are sine, cosine, tangent, cotangent, secant and cosecant.
Also, the above mnemonics can only apply to right angle triangles.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




