
How to derive the mode formula for grouped data?
Answer
499.8k+ views
Hint: To solve this question, we will first make 3 bar graphs of frequencies
Complete step-by-step answer:
Let us first define the mode for grouped data. The mode of a list of data values is simply the most common values (or the values if any). When the data are grouped as in a histogram, we will normally talk only about the modal class (the class, or group with the greatest frequency) because we don’t know the individual values. The derivation of the mode formula is given by using the bar graph.
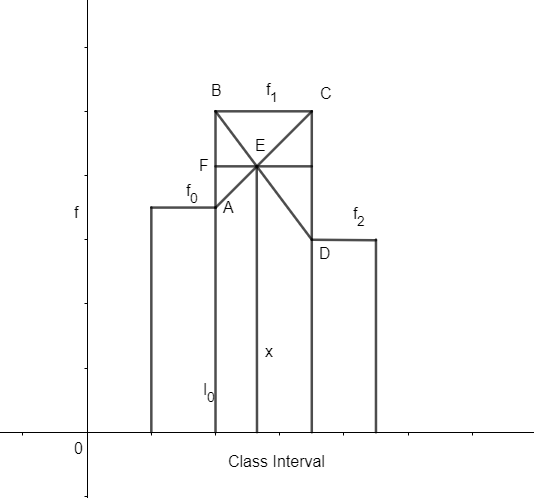
Let the frequency of the modal class be
The relative side ratio is also equal.
And BE is nothing but
Again we have
Clearly,
Therefore, we have,
We know that
Let, FE be x.
Therefore, the mode can be obtained by adding this value of x to
Substituting the value of x as obtained from above, we get,
Hence, the mode formula is determined.
Note: We have used the bar graph to determine the mode formula. So,
Complete step-by-step answer:
Let us first define the mode for grouped data. The mode of a list of data values is simply the most common values (or the values if any). When the data are grouped as in a histogram, we will normally talk only about the modal class (the class, or group with the greatest frequency) because we don’t know the individual values. The derivation of the mode formula is given by using the bar graph.

Let the frequency of the modal class be
The relative side ratio is also equal.
And BE is nothing but
Again we have
Clearly,
Therefore, we have,
We know that
Let, FE be x.
Therefore, the mode can be obtained by adding this value of x to
Substituting the value of x as obtained from above, we get,
Hence, the mode formula is determined.
Note: We have used the bar graph to determine the mode formula. So,
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




