
Derive the formula for height and area of an equilateral triangle.
Answer
511.8k+ views
Hint: We will first construct the figure of an equilateral triangle. As we know that equilateral triangles have sides of all equal length and equal angles of
Next, we have to derive the area of an equilateral triangle, we will use the basic formula of triangle that is
Complete step by step solution: We will first construct the figure of an equilateral triangle whose side is represented as
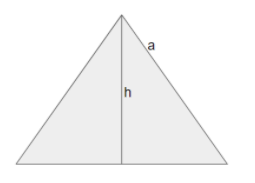
As we know that equilateral triangles have sides of all equal length and angles of
Now, as we have let the side of the original equilateral triangle as
Thus, from this, we get that
Which further gives us
Now, we will substitute it into the height of the triangle.
Thus, we get,
Next, we will derive the formula for the area of an equilateral triangle.
The sides of an equilateral triangle are shown by
As we know that the area of triangle is given by:
We already have the derived formula for the height of the triangle, and the base of the triangle is given as
Thus, we get,
Hence, we can conclude that the height is derived as
Note: Remember the basic formula for the area of the triangle. Do not get confused in the special triangle that is
Next, we have to derive the area of an equilateral triangle, we will use the basic formula of triangle that is
Complete step by step solution: We will first construct the figure of an equilateral triangle whose side is represented as

As we know that equilateral triangles have sides of all equal length and angles of
Now, as we have let the side of the original equilateral triangle as
Thus, from this, we get that
Which further gives us
Now, we will substitute it into the height of the triangle.
Thus, we get,
Next, we will derive the formula for the area of an equilateral triangle.
The sides of an equilateral triangle are shown by
As we know that the area of triangle is given by:
We already have the derived formula for the height of the triangle, and the base of the triangle is given as
Thus, we get,
Hence, we can conclude that the height is derived as
Note: Remember the basic formula for the area of the triangle. Do not get confused in the special triangle that is
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




