
Derive the expression for refractive index of the material of the prism in terms of angle of the prism and angle of minimum deviation.
Answer
444.6k+ views
9 likes
Hint: First draw a ray diagram indicating a ray of incidence and a ray of emergence. Then indicate all the necessary angles in the diagram. Then by using the conditions of minimum deviation derive an expression for the angle of incidence and angle of refraction. And the last stage would be the application of Snell’s law to get to the answer.
Formula used:
Snell’s law states that when a light wave passes through the interface of two different media, the ratio of sine of angle of incidence and refraction is a constant.
Mathematically,
Complete step-by-step answer:
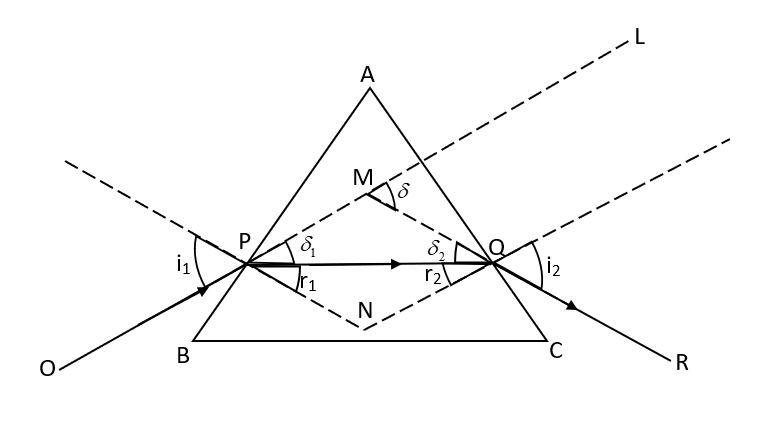
Let us consider a ray diagram for a prism in which OP is the ray of incidence which makes angle
According to the question, minimum deviation is taking place and we know that for minimum deviation,
And we know that
And,
Also,
Now, from Snell’s law, we can write that
This is the expression for refractive index.
Note: The conditions of minimum deviation are required in this question and should be applied correctly. Snell’s law for light waves passing through an interface of two media is also a key concept in determining the expression for the refractive index. An assumption has also been made here that the refractive index of the surrounding medium is 1.
Formula used:
Snell’s law states that when a light wave passes through the interface of two different media, the ratio of sine of angle of incidence and refraction is a constant.
Mathematically,
Complete step-by-step answer:

Let us consider a ray diagram for a prism in which OP is the ray of incidence which makes angle
According to the question, minimum deviation is taking place and we know that for minimum deviation,
And we know that
And,
Also,
Now, from Snell’s law, we can write that
This is the expression for refractive index.
Note: The conditions of minimum deviation are required in this question and should be applied correctly. Snell’s law for light waves passing through an interface of two media is also a key concept in determining the expression for the refractive index. An assumption has also been made here that the refractive index of the surrounding medium is 1.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




