
Construct the incircle and circumcircle of an equilateral
Answer
538.5k+ views
3 likes
Hint: Incentre is the meeting point of angle bisectors of triangle while circumcenter is meeting point of perpendicular bisector of sides and in equilateral triangle all the centers lie on the same point.
Complete step-by-step answer:
Here we have to construct the incircle and circumcircle of an equilateral
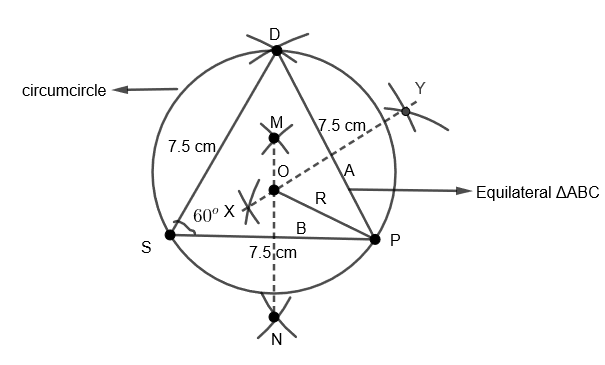
First of all we have to construct the equilateral
1. Draw the line
2. Now, with
3. With
4. Join
Now to draw the circumcenter of
1. As we know the circumcenter is a point of intersection of perpendicular bisectors of sides of a triangle. First, construct the perpendicular bisectors of sides.
2. We first have to mark the mid points of sides
3. Now with
4. With the same radius and center as
5. Now with
6. With the same radius and center as
7. The point of intersection of
8. Circumradius of the given circle is
9. Therefore, with
10. Now measure the circumradius that is the length of
Now to draw the incircle of
1. Incircle is the point of intersection of angle bisectors of triangles.
2. As we know that, in an equilateral triangle, all the centers coincide. Therefore, with

3. Inradius of the given incircle is
4. Now measure the inradius that is the length of
Therefore ratio radius of circumcircle to the radius of incircle
Therefore we get,
Note: Students can also first construct the angle bisectors and get the center with their intersection which is the same as
Complete step-by-step answer:
Here we have to construct the incircle and circumcircle of an equilateral

First of all we have to construct the equilateral
1. Draw the line
2. Now, with
3. With
4. Join
Now to draw the circumcenter of
1. As we know the circumcenter is a point of intersection of perpendicular bisectors of sides of a triangle. First, construct the perpendicular bisectors of sides.
2. We first have to mark the mid points of sides
3. Now with
4. With the same radius and center as
5. Now with
6. With the same radius and center as
7. The point of intersection of
8. Circumradius of the given circle is
9. Therefore, with
10. Now measure the circumradius that is the length of
Now to draw the incircle of
1. Incircle is the point of intersection of angle bisectors of triangles.
2. As we know that, in an equilateral triangle, all the centers coincide. Therefore, with

3. Inradius of the given incircle is
4. Now measure the inradius that is the length of
Therefore ratio radius of circumcircle to the radius of incircle
Therefore we get,
Note: Students can also first construct the angle bisectors and get the center with their intersection which is the same as
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




