
What is the center of the circle inscribed in a square formed by lines
(a). (4, 7)
(b). (7,4)
(c). (9,4)
(d). (4,9)
Answer
540.6k+ views
Hint: Solve the two equations to find the equation of four lines that form the square. Use the concept that the center of the square is the same as the center of the inscribed circle to find the center of the inscribed circle.
Complete step by step answer:
It is given that the square is formed by the following lines:
Let us solve equation (1) to get the equations of two lines.
We know that – 8x can be split as – 6x and – 2x, then, we have:
Now, we can group the common terms.
Hence, the equations of the two lines are x = 2 and x = 6.
We now solve equation (2) to find the equation of the other two lines which form the square.
We know that – 14y can be split into – 9y and – 5y, then, we have:
Taking the common terms and simplifying, we get:
Hence, the equations of the other two lines are y = 5 and y = 9.
Hence, the lines x =2, x= 6, y = 5 and y = 9 form a square.
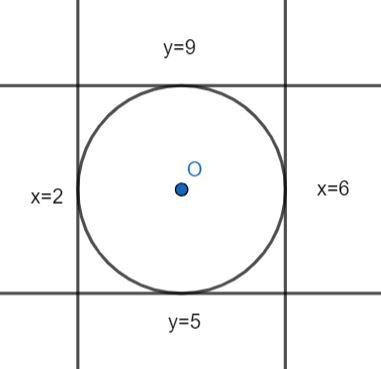
We know that the centre of the inscribed circle is the centre of the square itself.
The centre of the square is given as:
Hence, the centre of the inscribed circle is (4,7).
Note: You can also find the center of the inscribed circle by considering the distance of the center from any of the sides and using the equation of that respective side.
Complete step by step answer:
It is given that the square is formed by the following lines:
Let us solve equation (1) to get the equations of two lines.
We know that – 8x can be split as – 6x and – 2x, then, we have:
Now, we can group the common terms.
Hence, the equations of the two lines are x = 2 and x = 6.
We now solve equation (2) to find the equation of the other two lines which form the square.
We know that – 14y can be split into – 9y and – 5y, then, we have:
Taking the common terms and simplifying, we get:
Hence, the equations of the other two lines are y = 5 and y = 9.
Hence, the lines x =2, x= 6, y = 5 and y = 9 form a square.

We know that the centre of the inscribed circle is the centre of the square itself.
The centre of the square is given as:
Hence, the centre of the inscribed circle is (4,7).
Note: You can also find the center of the inscribed circle by considering the distance of the center from any of the sides and using the equation of that respective side.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




