
How do you calculate
Answer
484.5k+ views
Hint: We use the concept that arc means inverse of the function. We are given the value of sine of an angle. Use the trigonometric identity
* Arc cosine of a function is defined as the inverse cosine of the function. When
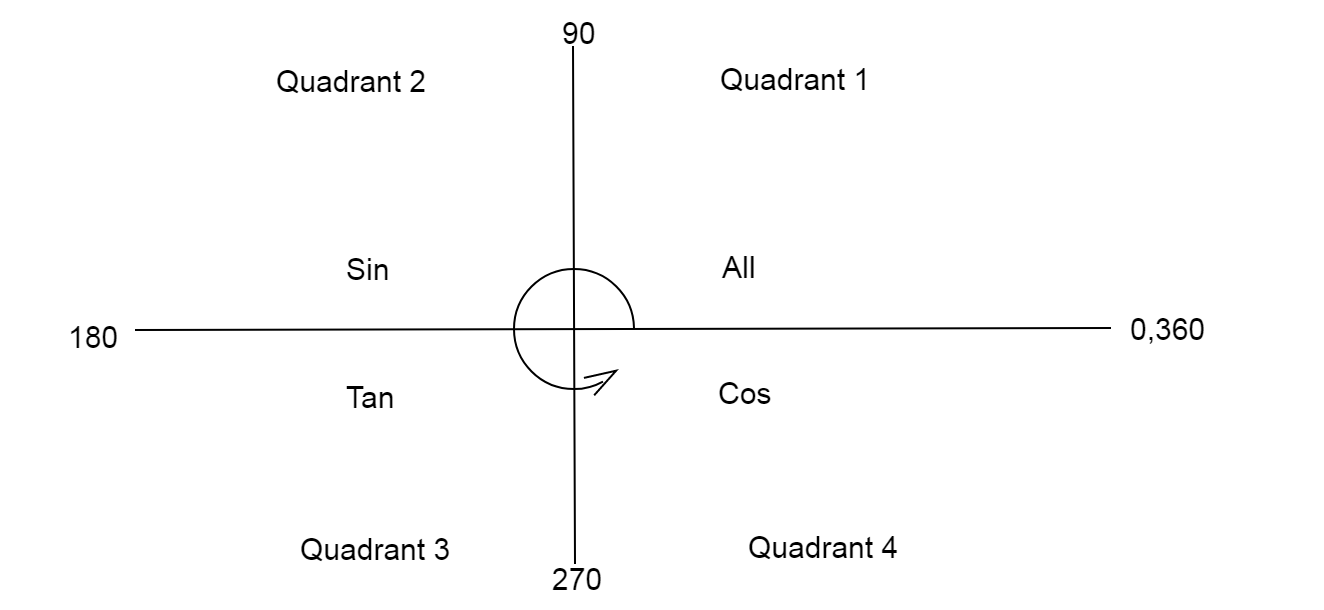
* We know the values of all trigonometric angles are positive in the first quadrant.
Values of only
Values of only
Values of only
Complete step-by-step answer:
Let us assume the angle as ‘x’
We are given that
Since we know that
Then we can write
Take cosine on both sides of the equation
Cancel cosine and cosine inverse on left side of the equation
We know that the value of
Also, we know cosine is an even function, so,
We can write
i.e.
i.e.
So equation (1) becomes
Taking inverse sine function on both sides
Cancel inverse of the function by the function
Note:
Many students make mistake of calculating the angle inside cosine as positive which is wrong, the value of cosine is given negative so we will have to look at the quadrant diagram and figure out what to add or subtract the angle from. Keep in mind we can use table for trigonometric terms if we don’t remember the values at some common angles
* Arc cosine of a function is defined as the inverse cosine of the function. When
* We know the values of all trigonometric angles are positive in the first quadrant.
Values of only
Values of only
Values of only

Complete step-by-step answer:
Let us assume the angle as ‘x’
We are given that
Since we know that
Then we can write
Take cosine on both sides of the equation
Cancel cosine and cosine inverse on left side of the equation
We know that the value of
Also, we know cosine is an even function, so,
We can write
i.e.
i.e.
So equation (1) becomes
Taking inverse sine function on both sides
Cancel inverse of the function by the function
Note:
Many students make mistake of calculating the angle inside cosine as positive which is wrong, the value of cosine is given negative so we will have to look at the quadrant diagram and figure out what to add or subtract the angle from. Keep in mind we can use table for trigonometric terms if we don’t remember the values at some common angles
| ANGLEFUNCTION | | | | | |
| Sin | 0 | | | | 1 |
| Cos | 1 | | | | 0 |
| Tan | 0 | | 1 | | Not defined |
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




