
Calculate the area of a circular ring whose outer and inner radii are
A.
B.
C.
D.
Answer
419.1k+ views
Hint: In this question, we need to find the area of a circular ring. A ring is nothing but a space between two concentric circles. Concentric circles are circles with the same centre and different radius. We can find the area of the ring by subtracting the area of the outer circle and area of the inner circle.
Formula used :
Where
Complete step by step answer:
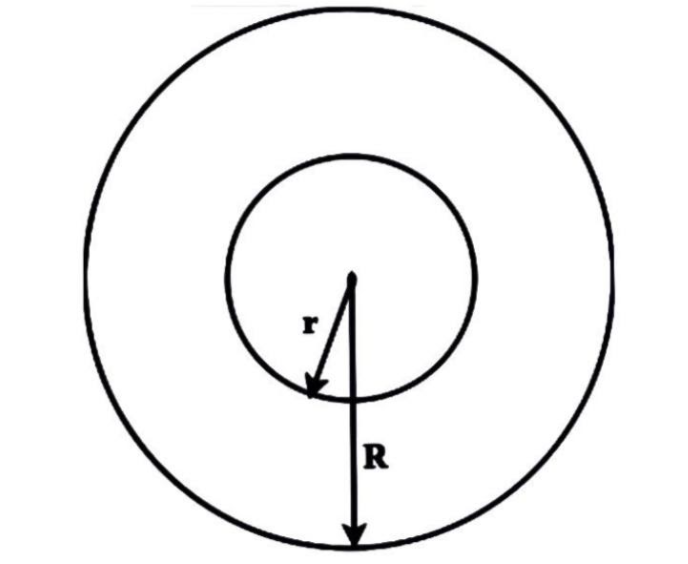
Given, outer and inner radii are
Let us consider the radius of the outer circle as
Radius of outer circle ,
Radius of inner circle,
We can find the area of the ring by subtracting the area of the outer circle and area of the inner circle.
⇒
First we can find the area of the outer circle with radius
By substituting the values,
We get,
By multiplying,
We get,
Now we can find the area of the inner circle with radius
By substituting the values,
We get,
By multiplying,
We get,
Now we can find the area of the ring,
By subtracting,
We get,
Thus we get the area of the circular ring is
So, the correct answer is “Option C”.
Note:
Alternative solution :
We can find the area of the ring by subtracting the area of the outer circle and area of the inner circle.
By taking
We get ,
By substituting the values,
We get,
By simplifying,
We get,
By subtracting,
We get,
By multiplying,
We get,
Thus we get the area of the circular ring as
We can also find the area of the circular ring in this method.
Formula used :
Where
Complete step by step answer:

Given, outer and inner radii are
Let us consider the radius of the outer circle as
Radius of outer circle ,
Radius of inner circle,
We can find the area of the ring by subtracting the area of the outer circle and area of the inner circle.
⇒
First we can find the area of the outer circle with radius
By substituting the values,
We get,
By multiplying,
We get,
Now we can find the area of the inner circle with radius
By substituting the values,
We get,
By multiplying,
We get,
Now we can find the area of the ring,
By subtracting,
We get,
Thus we get the area of the circular ring is
So, the correct answer is “Option C”.
Note:
Alternative solution :
We can find the area of the ring by subtracting the area of the outer circle and area of the inner circle.
By taking
We get ,
By substituting the values,
We get,
By simplifying,
We get,
By subtracting,
We get,
By multiplying,
We get,
Thus we get the area of the circular ring as
We can also find the area of the circular ring in this method.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Write examples of herbivores carnivores and omnivo class 10 biology CBSE

Difference between mass and weight class 10 physics CBSE

List out three methods of soil conservation

Leap year has days A 365 B 366 C 367 D 368 class 10 maths CBSE

How does Tommy describe the old kind of school class 10 english CBSE




